Study on the flow and densification behaviors of powder sintered steel with different carbon contents during clod compression
-
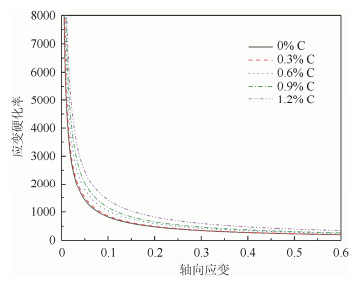
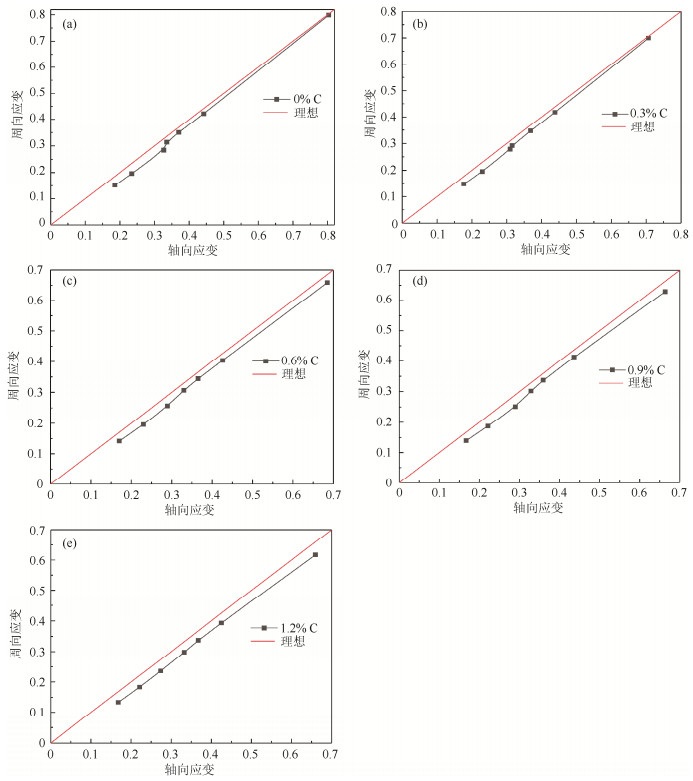
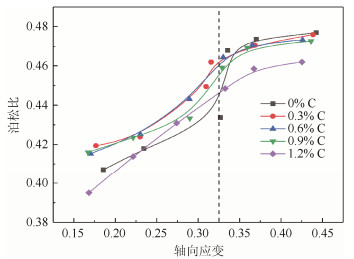
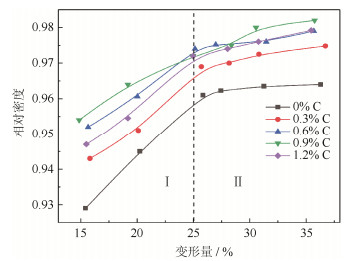
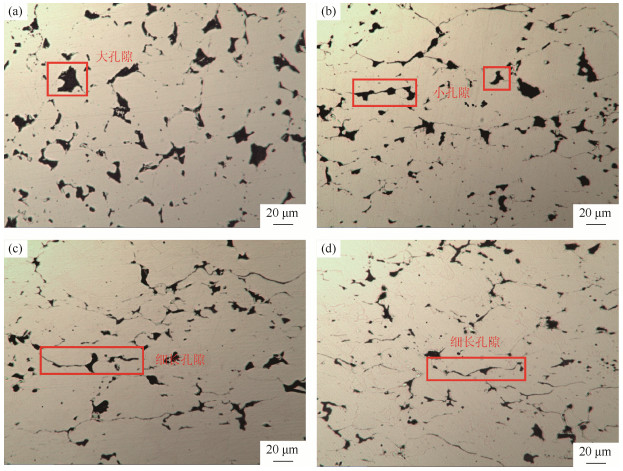
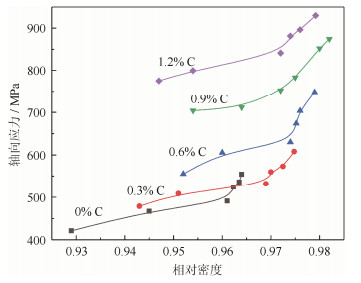
摘要: 在材料万能试验机上对碳含量（质量分数）为0%、0.3%、0.6%、0.9%、1.2%的粉末烧结钢进行冷压变形试验，利用Hollomon方程对粉末烧结钢流变应力数据进行非线性拟合，结合显微组织形貌分析烧结钢冷压流变致密化行为和孔隙、晶粒变形机制。结果表明：烧结钢冷压应变硬化行为符合Hollomon方程，随着碳含量的增加，极限断裂应变量逐渐减小；在相同应变下，随着碳含量的增加，烧结钢应变硬化率逐渐上升，致密化效果先增强后减弱，在碳质量分数为0.9%时最佳；随着应变增加，烧结钢孔隙闭合，晶粒由等轴状变为片状；烧结钢的流变致密化过程在低应变下以致密化和致密化硬化为主，在高应变下以变形和基体加工硬化为主。Abstract: The cold compression tests of the powder sintered steels with the carbon mass fraction of 0%, 0.3%, 0.6%, 0.9%, and 1.2% were carried out on the universal material testing machine. The tested stress?strain data were nonlinearly fitted by the Hollomon equation. The behaviors of flow and densification and the deformation mechanisms of pore and grain were analyzed, combining with the microstructure analysis of the powder sintered steels. The results show that, the cold compression strain hardening behavior of the sintered steels conforms to the Hollomon equation, and the ultimate fracture strain decreases with the increase of the carbon content. In the same strain condition, the strain hardening rate of the sintered steels increases gradually with the increase of carbon content, and the densification effect first increases and then decreases, showing the best when the carbon content is 0.9%. With the increase of strain, the pores of the sintered steels are gradually closed, and the grains change from the equiaxed crystal into the flaky structure. During the flow and densification processes, the densification and densification hardening are dominant under the low strains, while the deformation and the matrix work-hardening are dominant under the high strains.
-
Keywords:
- powder sintered steels /
- cold compression /
- flow deformation /
- densification
-
近代科学技术，特别是航空航天技术的发展，对所用工程材料性能提出了更高要求，如更高的比强度和比刚度，传统单一金属材料较难满足服役性能要求[1]。金属基复合材料既可保留金属材料的主要特性，又具有增强体的特性，有望满足高技术发展需求[2]。
金属基复合材料的研究始于20世纪60年代初期，80年代以来，美、日等国加大了对复合材料的研究开发，并采用粉末冶金法、熔铸技术、压力渗透技术等技术制备出性能优良的颗粒增强型铝基复合材料[3−4]。SiCp/Al复合材料由于轻质、高比强度、高比刚度、低热膨胀系数、良好的抗磨损等优点，被广泛用于航空航天、汽车、电子通讯、军事等领域。15%～20%SiCp/Al复合材料（体积分数）作为结构材料被应用于承载飞机上的承力结构件与电子元器件[5−6]。美国、日本、印度等国把SiCp/Al复合材料应用到汽车用连杆和缸套上[7−9]。我国于1981年启动金属基复合材料研究，多种复合材料产品在尖端国防领域得到应用，随着军民技术一体化需求的急剧增长，对金属基复合材料的研究更加迫切。
复合材料在制备过程中需要二次加工变形，国内外学者对铝合金和低体积分数SiCp/Al复合材料的研究较多[10−11]。Shao等[12]认为细小的SiCp和细小的2024Al粉末导致大量晶界的存在，使动态再结晶区域向低温和高应变速率区域移动，并且使得功率耗散效率系数的峰值减小。Rajamuthamil selvan和Ramanathan[13]研究了SiC颗粒体积分数对复合材料热变形行为的影响，发现SiC颗粒含量增多对复合材料的再结晶有促进作用。
材料固有的加工性与材料的化学组成、变形条件和变形量有关[14]。随着颗粒体积分数增加，加工难度增大，因此对中高体积分数（30%～60%）颗粒增强铝基复合材料热变形行为展开研究十分有意义。40%SiCp/Al复合材料（体积分数）作为一种新的结构材料有着广阔的发展前景，虽可以进行挤压、轧制和锻造等传统的热塑性变形加工，但可加工性能显着下降，要实现产业化仍需做大量的研究。以动态材料模型为基础的加工图和热变形本构方程是材料加工设计和优化的一种有效手段，已经得到了广泛应用[15−16]。本文在不同变形条件下对40%SiCp/Al复合材料（体积分数）进行等温热压缩实验研究，分析材料的热加工变形行为特征，并找出影响规律，提出优化加工参数，为优化该复合材料的热加工工艺提供指导。
1. 实验材料及方法
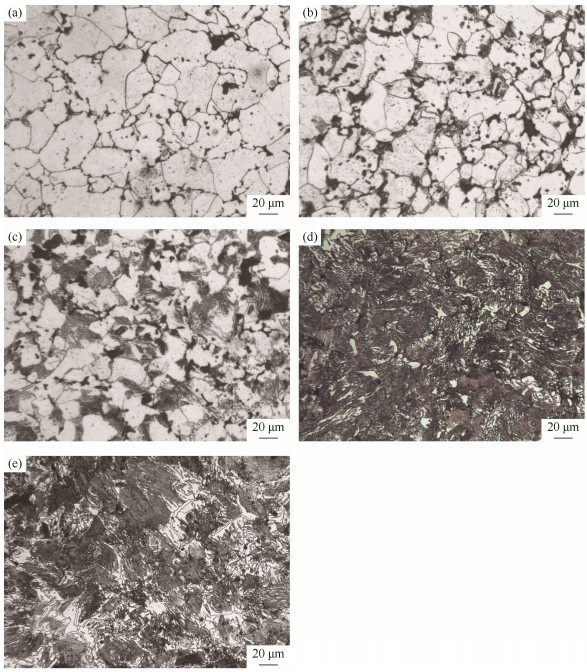
实验材料为碳化硅颗粒增强铝基复合材料（40%SiCp/Al，体积分数)。以平均直径10 μm的2024Al粉末为基体材料，增强体SiC颗粒粒径为15 μm，通过球磨混粉、冷压和热压烧结而成，复合材料的微观组织如图1所示。利用线切割将烧结后的试样加工成8 mm×12 mm的热模拟试样，用于等温热压缩变形。采用圆柱体单向压缩法，变形设备为Gleeble-1500D热模拟机，加热速率为10 ℃/s，变形温度分别为350、400、450和500 ℃，应变速率分别为0.01、0.10、1.00和10.00 s−1。压缩变形终了立即水淬，以便保留高温微观组织。总压缩量达到应变值0.7，即总变形程度为50%左右。在热变形过程中，在试样两端填充润滑剂以减少摩擦的影响。热模拟系统自动采集应力、应变、温度等数据。
2. 结果及分析
2.1 真应力-应变曲线
图2为40%SiCp/2024A1复合材料热变形过程的真应力-应变曲线。由图可见，在热变形过程中，当应变超过一定值后，复合材料呈现稳态流变特征，应变增加时真应力改变很小。在应变速率保持一定的情况下，随应变逐渐增加，流变应力先快速升高达到峰值，然后逐渐小幅下降，进入近似稳态流变状态。整体来看，稳态流变应力随变形温度升高而逐渐减小。在变形温度一定时，流变应力随应变速率的增大而增大，表明该复合材料有正的应变速率敏感性，即应变速率越大，复合材料实现稳态变形就越困难。图2（d）中显示复合材料在高应变速率变形时，应力-应变曲线上出现明显的连续波浪峰，表现出应力不连续屈服现象，这可能是由于材料发生动态再结晶和动态失效或者局部流变而引起的。
![]()
图 2 不同应变速率下40%SiCp/Al复合材料的真应力-应变曲线：（a）0.01 s−1；（b）0.10 s−1；（c）1.00 s−1；（d）10.00 s−1Figure 2. True stress-strain curves of the 40%SiCp/Al composites at different strain rates: (a) 0.01 s−1; (b) 0.10 s−1; (c) 1.00 s−1; (d) 10.00 s−12.2 构建复合材料本构方程
基于模拟系统自动采集的应力、应变、温度等数据建立本构方程。当应力较低时如式（1）所示，当应力较高时如式（2）所示。
$$ \dot \varepsilon = {A_1}\exp \left( {\beta \sigma } \right)\exp \left( { - \frac{Q}{{RT}}} \right) $$ (1) $$ \dot \varepsilon = {A_2}{\sigma ^{{n_1}}}\exp \left( { - \frac{Q}{{RT}}} \right) $$ (2) 式中：
$ \dot \varepsilon $ 为应变速率，s−1；σ为流变应力，MPa；ε为应变；A1、A2、n1、β为材料常数；Q为变形激活能，kJ·mol−1；R为气体常数；T为变形温度，K。Sellars和Tegart提出式（3）适用于所有应力。Zener和Hollomon为更好地描述材料的热加工行为提出了Zener-Hollomon参数（Z参数），如式（4）所示。
$$ \dot \varepsilon = A{\left[ {\sinh \left( {\alpha \sigma } \right)} \right]^n}\exp \left( { - \frac{Q}{{RT}}} \right) $$ (3) $$ Z = \dot \varepsilon \exp \left( {\frac{Q}{{RT}}} \right) = A{\left[ {\sinh \left( {\alpha \sigma } \right)} \right]^n} $$ (4) 式中：A、α、n为材料常数。
为求解热变形激活能（Q），可以对式（1）～式（3）同时取对数，绘制线性方程。在高应力水平下，如式（5）所示，在低应力水平下，如式（6）所示，对所有应力水平，如式（7）所示。
$$ \ln \dot \varepsilon = \ln {A_1} + \beta \sigma - {Q \mathord{\left/ {\vphantom {Q {\left( {RT} \right)}}} \right. } {\left( {RT} \right)}} $$ (5) $$ \ln \dot \varepsilon = \ln {A_2} + {n_1}\ln \sigma - {Q \mathord{\left/ {\vphantom {Q {\left( {RT} \right)}}} \right. } {\left( {RT} \right)}} $$ (6) $$ \ln \dot \varepsilon = \ln A - {Q \mathord{\left/ {\vphantom {Q {\left( {RT} \right) + n\ln }}} \right. } {\left( {RT} \right) + n\ln }}\left[ {\sinh \left( {\alpha \sigma } \right)} \right] $$ (7) 对式（3）求偏导可得式（8）。为了求解本构方程，需求得材料常数n1、n、β、M值，其中ln
$ \dot \varepsilon $ -lnσ拟合曲线斜率是n1值，ln$ \dot \varepsilon $ -σ拟合曲线斜率是β值，ln[sinh(ασ)]−1/T斜率是M值，ln$ \dot \varepsilon $ -ln[sinh(ασ)]斜率平均值是n值。$$\begin{split} &Q = R\left\{ {{{\partial \ln \left[ {\sinh \left( {\alpha \sigma } \right)} \right]} \mathord{\left/ {\vphantom {{\partial \ln \left[ {\sinh \left( {\alpha \sigma } \right)} \right]} {\partial \left( {{1 \mathord{\left/ {\vphantom {1 T}} \right. } T}} \right)}}} \right. } {\partial \left( {{1 \mathord{\left/ {\vphantom {1 T}} \right. } T}} \right)}}} \right\} \cdot\\ &\qquad {\left\{ {{{\partial \ln \varepsilon } \mathord{\left/ {\vphantom {{\partial \ln \varepsilon } {\partial \ln \left[ {\sinh \left( {\alpha \sigma } \right)} \right]}}} \right. } {\partial \ln \left[ {\sinh \left( {\alpha \sigma } \right)} \right]}}} \right\}_T} = RnM\end{split} $$ (8) 将不同变形条件下峰值应力（σp，MPa）以及对应的
$ \dot \varepsilon $ 带入式（5）～式（7），可以绘制图3～图5，图中直线斜率的平均值即为本构方程中所求材料常数值，即M、n1、n、β，其中α=β/n1![]()
图 3 真应变为0.1时应力、应变速率和温度关系：（a）ln$ \dot \varepsilon $ -σ；（b）ln$ \dot \varepsilon $ -lnσ；（c）ln$ \dot \varepsilon $ -ln[sinh(ασ)]；（d）ln[sinh(ασ)]-1/TFigure 3. Relationship between stress, stress rate, and temperature at true strain of 0.1: (a) ln$ \dot \varepsilon $ -σ; (b) ln$ \dot \varepsilon $ -lnσ; (c) ln$ \dot \varepsilon $ -ln[sinh(ασ)]; (d) ln[sinh(ασ)]-1/T![]()
图 4 真应变为0.3时应力、应变速率和温度关系：（a）ln$ \dot \varepsilon $ -σ；（b）ln$ \dot \varepsilon $ -lnσ；（c）ln$ \dot \varepsilon $ -ln[sinh(ασ)]；（d）ln[sinh(ασ)]-1/TFigure 4. Relationship between stress, stress rate, and temperature at true strain of 0.3: (a) ln$ \dot \varepsilon $ -σ; (b) ln$ \dot \varepsilon $ -lnσ; (c) ln$ \dot \varepsilon $ -ln[sinh(ασ)]; (d) ln[sinh(ασ)]-1/T![]()
图 5 真应变为0.5时应力、应变速率和温度关系：（a）ln$ \dot \varepsilon $ -σ；（b）ln$ \dot \varepsilon $ -lnσ；（c）ln$ \dot \varepsilon $ -ln[sinh(ασ)]；（d）ln[sinh(ασ)]-1/TFigure 5. Relationship between stress, stress rate, and temperature at true strain of 0.5: (a) ln$ \dot \varepsilon $ -σ; (b) ln$ \dot \varepsilon $ -lnσ; (c) ln$ \dot \varepsilon $ -ln[sinh(ασ)]; (d) ln[sinh(ασ)]-1/T将得到的n和M数据代入式（4）和式（8），可以求得不同真应力的Q值和Z值，再由lnZ-ln[sinh(ασ)]曲线计算得到截距lnA值，如图6和表1所示。
![]()
图 6 不同真应变线下lnZ与ln[sinh(ασ)]关系：（a）0.1；（b）0.3；（c）0.5Figure 6. Relationship between lnZ and ln[sinh(ασ)] at the different true strain: (a) 0.1; (b) 0.3; (c) 0.5表 1 不同应变量时材料常数计算结果Table 1. Calculation results of the material constants at the different strainsε β n1 n M α / MPa−1 lnA Q / (kJ·mol−1) 0.1 0.134408 8.973713 6.656257 2882.649 0.015438 24.52115 157.288 0.3 0.133552 8.387507 6.149667 3088.224 0.016263 24.97749 157.903 0.5 0.134407 8.973715 6.601892 2865.210 0.015245 24.62439 157.273 根据表1可知复合材料常数的求解结果，将其代入式（3）和式（4）式可得本构方程。真应变为0.1时，变形激活能Q=157.288 kJ·mol−1，应力指数n=6.56，应力水平参数α=0.0154 MPa−1，结构因子A=4.46×1010。将上述材料参数代入式（3）和式（4），可得复合材料在真应力为0.1时的本构方程，如式（9）所示。
$$ \left\{ \begin{aligned} & {\dot \varepsilon = 4.46 \times {{10}^{10}}{{\left[ {\sinh \left( {0.0154\sigma } \right)} \right]}^{6.56}}\exp \left( { - \frac{{157.288}}{{RT}}} \right)} \\ & {Z = 4.46 \times {{10}^{10}}{{\left[ {\sinh \left( {0.0154\sigma } \right)} \right]}^{6.56}}{\text{ }}} \end{aligned} \right. $$ (9) 真应变为0.3时，变形激活能Q=157.903 kJ·mol−1，应力指数n=6.14，应力水平参数α=0. 0162 MPa−1，结构因子A=7.04×1010。将上述材料参数代入式（3）和式（4），可得复合材料在真应力为0.3时的本构方程，如式（10）所示。
$$ \left\{ \begin{aligned} & {\dot \varepsilon = 7.04 \times {{10}^{10}}{{\left[ {\sinh \left( {0.0162\sigma } \right)} \right]}^{6.14}}\exp \left( { - \frac{{157.903}}{{RT}}} \right)} \\ & {Z = 7.04 \times {{10}^{10}}{{\left[ {\sinh \left( {0.0162\sigma } \right)} \right]}^{6.14}}{\text{ }}} \end{aligned} \right. $$ (10) 真应变为0.5时，变形激活能Q=157.273 kJ·mol−1，应力指数n=6.60，应力水平参数α=0. 0152 MPa−1，结构因子A=4.95×1010。将上述材料参数代入式（3）和式（4），可得复合材料在真应力为0.5时的本构方程，如式（11）所示。
$$ \left\{ \begin{aligned} & {\dot \varepsilon = 4.95 \times {{10}^{10}}{{\left[ {\sinh \left( {0.0152\sigma } \right)} \right]}^{6.60}}\exp \left( { - \frac{{157.273}}{{RT}}} \right)} \\ & {Z = 4.95 \times {{10}^{10}}{{\left[ {\sinh \left( {0.0152\sigma } \right)} \right]}^{6.60}}{\text{ }}} \end{aligned} \right. $$ (11) 复合材料热变形时应力的计算值与实验值如图7所示。由图可知，计算值与实验值比较接近，证明本构方程能较好地描述本复合材料的热变形力学行为。如图所示，不同应变条件下的变形激活能变化不大，复合材料变形激活能通常与变形抗力的变化相关，说明变形量增加时，变形抗力变化不大。
![]()
图 7 不同真应变下实验值与计算值分析与对比：（a）0.1；（b）0.3；（c）0.5Figure 7. Analysis and comparison of the experimental and calculated values at the different true strain: (a) 0.1; (b) 0.3; (c) 0.52.3 二维加工图
动态材料模型将复合材料的热加工过程看作一个消耗能量过程。如式（12）所示，输入功率（p）的耗散来源于两个方面：由材料塑性变形引起的耗散量（G）和由组织变化引起的功率耗散（J），其动态本构方程如式（13）所示。由式（12）和式（13）可得到式（14）和式（15）。
$$ p = \sigma \dot \varepsilon = G + J = \int_0^{\dot \varepsilon } \sigma {\text{d}}\dot \varepsilon + \int_0^\sigma {\dot \varepsilon } {\text{d}}\sigma $$ (12) $$ \sigma = K\dot \varepsilon $$ (13) $$ J = p - G = \sigma \dot \varepsilon - \int_0^{\dot \varepsilon } \sigma {\text{d}}\dot \varepsilon $$ (14) $$ J = \sigma \dot \varepsilon - \int_0^{\dot \varepsilon } {K{{\dot \varepsilon }^m}} {\text{d}}\dot \varepsilon = \frac{m}{{m + 1}}\sigma \dot \varepsilon $$ (15) 式中：m为材料的应变速率敏感指数。材料处于理想耗散状态时，m=1，J达到最大值，如式（16）所示。此时引入功率耗散效率系数（η），如式（17）所示，可以看出功率耗散效率系数与应变速率敏感指数直接相关。
$$ {J_{\max }} = J\left( {m = 1} \right) = \sigma \dot \varepsilon /2 $$ (16) $$ \eta = \frac{J}{{{J_{\max }}}} = \frac{{2m}}{{m + 1}} $$ (17) 在应变速率和温度所构成的二维平面上绘出等功率耗散效率系数曲线即为功率耗散图。按照动态材料模型原理及Prasad失稳判断准则，耗散函数同应变速率满足式（18），将式（17）代入可最终化简得到式（19）。
$$ \frac{{{\text{d}}J}}{{{\text{d}}\dot \varepsilon }} < \frac{J}{{\dot \varepsilon }} $$ (18) $$ \frac{{\partial \ln \left( {\dfrac{m}{{m + 1}}} \right)}}{{\partial \ln \dot \varepsilon }} + m < 0 $$ (19) 此处定义材料的流变失稳条件（ξ(
$ \dot \varepsilon $ )）如式（20）所示，可以看到失稳条件与应变速率敏感指数有关。$$ \xi \left( {\dot \varepsilon } \right) = \frac{{\partial \ln \left( {\dfrac{m}{{m + 1}}} \right)}}{{\partial \ln \dot \varepsilon }} + m < 0 $$ (20) 真应变为0.1，0.3和0.5时的功率耗散图、失稳图及二维加工图分别如图8、图9、图10所示。从图8（a）可知，应变为0.1时，功率耗散效率系数值在365～420 ℃区域最大，约为0.21；从图9（a）可知，应变为0.3时，功率耗散效率系数值在377～420 ℃区域最大，约为0.22；从图10（a）可知，应变为0.5时，功率耗散效率系数值在379～420 ℃区域最大，约为0.27。在功率耗散效率系数值最大区域很大可能发生了组织转变。从图8（b）可知，应变为0.1时，失稳区域主要为温度350～430 ℃，应变速率为0.03～9.97 s−1；从图9（b）可知，应变为0.3时，失稳区域主要为温度350～432 ℃，应变速率为0.04～9.97 s−1；从图10（b）可知，应变为0.5时，失稳区域主要为温度350～439 ℃，应变速率为0.03～9.97 s−1。从图8（c）可知，温度433～490 ℃、应变速率0.03～9.97 s−1是该应变下最理想的加工区域；从图9（c）可知，温度436～490 ℃、应变速率0.04～9.97 s−1是该应变下最理想的加工区域；从图10（c）可知，温度440～492 ℃、应变速率0.04～9.97 s−1是该应变下最理想的加工区域。由此可知，在同一应变速率下，随温度的升高，功率耗散效率系数先增大后减小再增大。在350～430 ℃，功率耗散效率系数随应变速率的增大先增大后减小；在430～500 ℃，功率耗散效率系数随应变速率的增大而增大。
![]()
图 8 应变为0.1时复合材料功率耗散图（a）、失稳图（b）和二维加工图（c）Figure 8. Power dissipation diagram (a), instability diagram (b), and two-dimensional processing map (c) of the composites at the strain of 0.1![]()
图 9 应变为0.3时复合材料功率耗散图（a）、失稳图（b）和二维加工图（c）Figure 9. Power dissipation diagram (a), instability diagram (b), and two-dimensional processing map (c) of the composites at the strain of 0.3![]()
图 10 应变为0.5时复合材料功率耗散图（a）、失稳图（b）和二维加工图（c）Figure 10. Power dissipation diagram (a), instability diagram (b), and two-dimensional processing map (c) of the composites at the strain of 0.5由图8（a）、图9（a）和图10（a）可以看出，功率耗散效率系数随应变的增加而增大。由图8（b）、图9（b）、图10（b）可以看出应变对失稳区域影响不大。由图8（c）、图9（c）、图10（c）可以看出应变对理想加工区域影响不大。
3. 结论
（1）40%SiCp/Al复合材料应变速率和变形温度对流变应力有明显的影响，应变速率越大，变形温度越低，复合材料越难于达到稳态变形。
（2）40%SiCp/Al复合材料应变速率增大，流变应力升高；变形温度升高，流变应力降低。热压缩变形时的流变行为可采用Zener-Hollomon参数的双曲正弦形式来描述。
（3）应变（真应变从0.1到0.5）对变形激活能影响不大，变形量增加，受到的变形抗力基本不变。
（4）在变形过程中，SiCp/Al复合材料容易发生破坏的区域主要分布在中低温范围。应变量变化对失稳区域影响不大。复合材料能够稳定变形的区域在中高温、低应变速率区，建议最佳加工区域温度为436～491 ℃，应变速率为0.04～9.97 s−1。
-
![]()
图 1 不同质量分数碳含量试样原始组织：（a）0%；（b）0.3%；（c）0.6%；（d）0.9%；（e）1.2%
Figure 1. Original microstructures of the specimens with the different carbon mass fractions: (a) 0%; (b) 0.3%; (c) 0.6%; (d) 0.9%; (e) 1.2%
![]()
图 2 粉末烧结钢试样孔隙形貌和显微组织观察区示意图
Figure 2. Observation area diagram of the pore images and the microstructures in the powder sintered steel specimens
![]()
图 3 粉末烧结钢在不同变形量下冷压真应力–真应变曲线
Figure 3. True stress-true strain curves of the powder sintered steels by cold compression at the different deformation
![]()
图 4 粉末烧结钢应变硬化率–应变曲线
Figure 4. Strain hardening rate-strain curves of the powder sintered steels
![]()
图 5 含碳质量分数为0.3%的冷压粉末烧结钢在不同变形量下的晶粒形貌: (a)15%；(b)25%；(c)35%；(d)极限应变
Figure 5. Grain morphology of the powder sintered steels by cold compression at the different deformation with the carbon mass fraction of 0.3%: (a) 15%; (b) 25%; (c) 35%; (d) ultimate strain
![]()
图 6 不同碳含量烧结钢的轴向应变与周向应变的关系：（a）0%；（b）0.3%；（c）0.6%；（d）0.9%；（e）1.2%
Figure 6. Relationship between the axial strain and the circumferential strain of the powder sintered steels with the different carbon mass fractions: (a) 0%; (b) 0.3%; (c) 0.6%; (d) 0.9%; (e) 1.2%
![]()
图 7 不同碳含量烧结钢的轴向应变与泊松比的关系
Figure 7. Relationship between the axial strain and the poisson's ratio of the powder sintered steels with the different carbon mass fractions
![]()
图 8 不同碳含量烧结钢的变形量与相对密度的关系
Figure 8. Relationship between the deformation and relative density of the powder sintered steels with the different carbon mass fractions
![]()
图 9 含碳质量分数为0.3%的冷压烧结钢不同变形量下的孔隙形貌：(a)0%；(b)15%；(c)25%；(d)35%
Figure 9. Pore images of the powder sintered steels by cold compression with the carbon mass fraction of 0.3% at the different deformation: (a) 0%; (b) 15%; (c) 25%; (d) 35%
![]()
图 10 不同碳含量粉末烧结钢试样冷压变形量为15%时的孔隙形貌: （a）0%；（b）0.3%；（c）0.6%；（d）0.9%；（e）1.2%
Figure 10. Pore images of the powder sintered steels by cold compression with the different carbon mass fraction at the deformation of 15%: (a) 0%; (b) 0.3%; (c) 0.6%; (d) 0.9%; (e) 1.2%
![]()
图 11 不同碳含量粉末烧结钢试样相对密度与轴向应力的关系
Figure 11. Relationship between the relative density and the axial stress of the powder sintered steels with the different carbon mass fraction
表 1 Hollomon方程数值拟合结果
Table 1 Numerical fitting results of Hollomon equation
碳质量分数/ % K n 相关系数，R2 0.0 638.11 0.209 0.96391 0.3 694.29 0.203 0.96552 0.6 821.03 0.199 0.97736 0.9 982.54 0.192 0.97821 1.2 1080.35 0.189 0.97948 
下载: 导出CSV
-
[1] 陈梦婷, 石建军, 陈国平. 粉末冶金发展状况. 粉末冶金工业, 2017, 27(4): 66 https://www.cnki.com.cn/Article/CJFDTOTAL-FMYG201704018.htm Chen M T, Shi J J, Chen G P. Development of powder metallurgy. Powder Metall Ind, 2017, 27(4): 66 https://www.cnki.com.cn/Article/CJFDTOTAL-FMYG201704018.htm
[2] Whittaker D. PM structural parts move to higher density and performance. Powder Metall, 2007, 50(2): 99 DOI: 10.1179/174329007X209114
[3] Akash G, Kandavel T K, Sai Kishan I, et al. Experimental investigations on deformation, densification and mechanical properties of sintered Fe–C–Mn low alloy P/M steels under hot upsetting. Mater Today, 2018, 5(8): 16073 http://www.sciencedirect.com/science/article/pii/s2214785318310423
[4] Gupta G K, Patel K K, Purohit R, et al. Effect of rolling on Ni–Ti–Fe shape memory alloys prepared through novel powder metallurgy route. Mater Today, 2017, 4(4): 5385 http://www.sciencedirect.com/science/article/pii/S2214785317307587
[5] Issa H K, Taherizadeh A, Maleki A, et al. Development of an aluminum/amorphous nano-SiO2 composite using powder metallurgy and hot extrusion processes. Ceram Int, 2017, 43(17): 14582 DOI: 10.1016/j.ceramint.2017.06.057
[6] Kumar D R, Loganathan C, Narayanasamy R. Effect of glass in aluminum matrix on workability and strain hardening behavior of powder metallurgy composite. Mater Des, 2011, 32(4): 2413 DOI: 10.1016/j.matdes.2010.12.008
[7] 黄培云. 粉末冶金原理. 2版. 北京: 冶金工业出版社, 2004 Huang P Y. Theory of Power Metallurgy. 2nd Ed. Beijing: Metallurgical Industry Press, 2004
[8] Haynes R. Development of sintered low alloy steels. Powder Metall, 1989, 32(2): 140 DOI: 10.1179/pom.1989.32.2.140
[9] 任学平, 王尔德, 霍文灿. 粉末体的屈服准则. 粉末冶金技术, 1992, 10(1): 8 https://www.cnki.com.cn/Article/CJFDTOTAL-FMYJ199201002.htm Ren X P, Wang E D, Huo W C. The yield criterion for powder compact. Powder Metall Technol, 1992, 10(1): 8 https://www.cnki.com.cn/Article/CJFDTOTAL-FMYJ199201002.htm
[10] 薛勇, 张治民, 张福祥, 等. 铝钨粉末合金挤压成形与本构模型的建立. 塑性工程学报, 2015, 22(2): 111 https://www.cnki.com.cn/Article/CJFDTOTAL-SXGC201502020.htm Xue Y, Zhang Z M, Zhang F X, et al. Extrusion forming of aluminum tungsten powder alloy and establishment of constitutive model. J Plast Eng, 2015, 22(2): 111 https://www.cnki.com.cn/Article/CJFDTOTAL-SXGC201502020.htm
[11] 胡建召. W–Cu20粉末板材轧制过程数值模拟与实验验证[学位论文]. 哈尔滨: 哈尔滨工业大学, 2017 Hu J Z. Numerical Simulation and Experimental Verification of Sheet Rolling Process of W–Cu20 Powders [Dissertation]. Harbin: Harbin Institute of Technology, 2017
[12] Venkata Kondaiah E, Kumaran S, Sundarrajan S. Study on densification behaviour of sintered AISI 4135 steel through hot upset forging. Mater Today, 2018, 5: 6543 http://www.sciencedirect.com/science/article/pii/S2214785317325774
[13] 李永志. 多孔烧结材料锻造镦粗成形致密的特性研究. 锻压技术, 2006, 31(1): 6 https://www.cnki.com.cn/Article/CJFDTOTAL-DYJE200601011.htm Li Y Z. Study on the deformation and densification of sintered powder upsetting. Forg Stamp Technol, 2006, 31(1): 6 https://www.cnki.com.cn/Article/CJFDTOTAL-DYJE200601011.htm
[14] Liu X, Xiao Z Y, Guan H J, et al. Experimental study on the surface densification of Fe–2Cu–0.6C powder metallurgy material. Mater Manuf Processes, 2016, 31(12): 1621 DOI: 10.1080/10426914.2015.1117619
[15] Vishnuraj J T, Kandavel T K, Sai Kishan I, et al. A study on deformation and densification characteristics of P/M Fe–C–Mn alloy steels under cold upset. Mater Today, 2018, 5: 16740 http://www.sciencedirect.com/science/article/pii/S2214785318311453
[16] Narayanasamy R, Pandey K S. Salient features in the cold upset-forming of sintered aluminium–3.5% alumina powder composite performs. J Mater Process Technol, 1997, 72(2): 201 DOI: 10.1016/S0924-0136(97)00169-6
[17] Narayanasamy R, Pandey K S. A study on the barrelling of sintered iron preforms during hot upset forging. J Mater Process Technol, 2000, 100(1): 87 http://www.sciencedirect.com/science/article/pii/S0924013699004574
[18] Narayanasamy R, Senthilkumar V, Pandey K.S. Effect of titanium carbide particle addition on the densification behavior of sintered P/M high strength steel preforms during cold upset forming. Mater Sci Eng A, 2007, 456: 180 DOI: 10.1016/j.msea.2006.11.118
[19] Hollomon J H. The effect of heat treatment and carbon content on the work hardening characteristics of several steels. Trans ASM, 1994, 32: 123 http://www.researchgate.net/publication/284222180_The_effect_of_heat_treatment_and_carbon_content_on_the_work_hardening_characteristics_of_several_steel
[20] 王从曾. 材料性能学. 北京: 北京工业大学出版社, 2001 Wang C Z. Material Properties. Beijing: Beijing University of Technology Press, 2001
[21] 刘智恩. 材料科学基础. 4版. 西安: 西北工业大学出版社, 2013 Liu Z E. Fundamentals of Material Science. 4th Ed. Xi'an: Northwest Polytechnic University Press, 2013
-
期刊类型引用(0)
其他类型引用(1)
计量
- 文章访问数: 462
- HTML全文浏览量: 188
- PDF下载量: 24
- 被引次数: 1
