Elevated temperature tensile deformation behavior of powder metallurgy Fe−2Cu−0.5C steels
-
摘要:
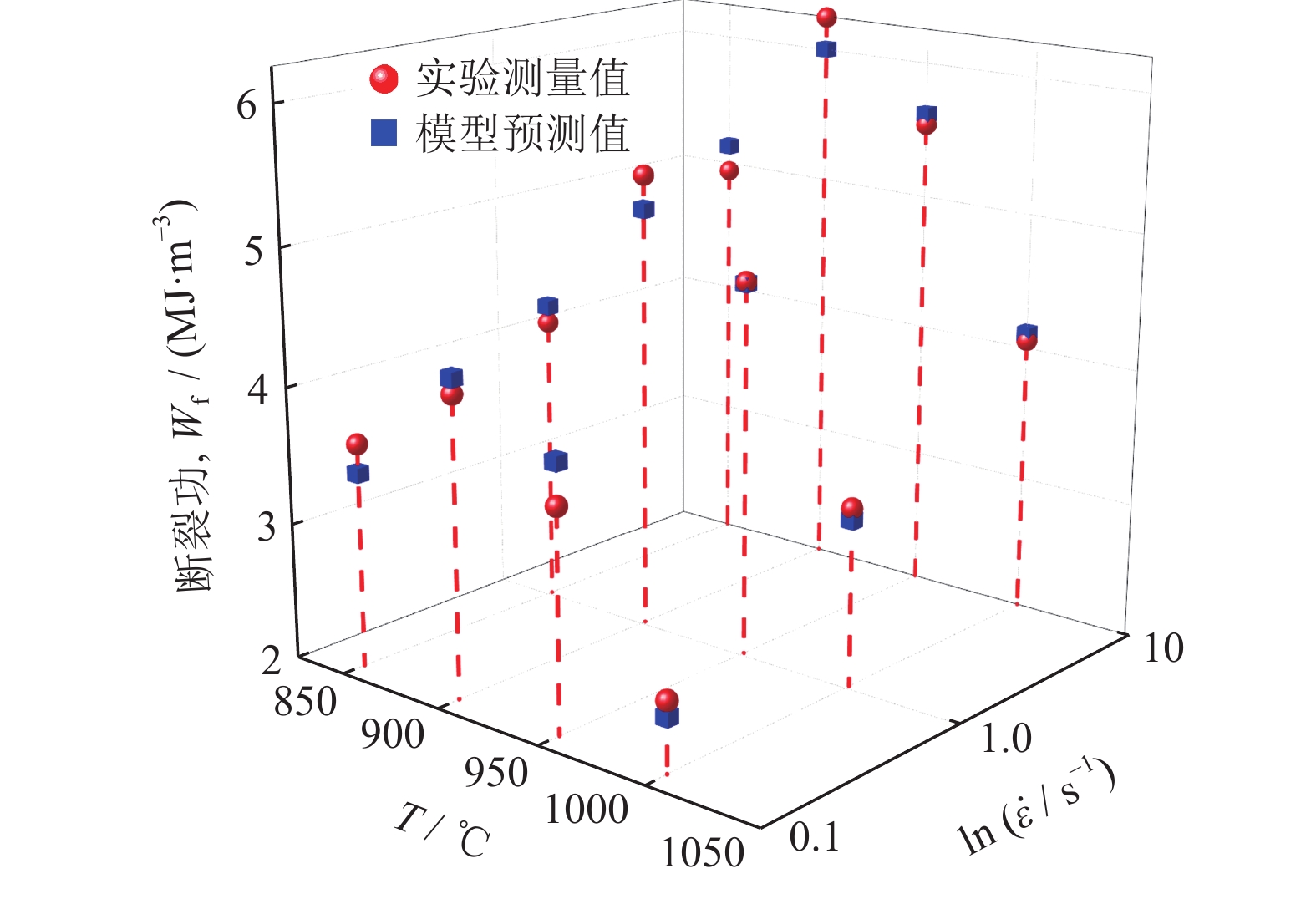
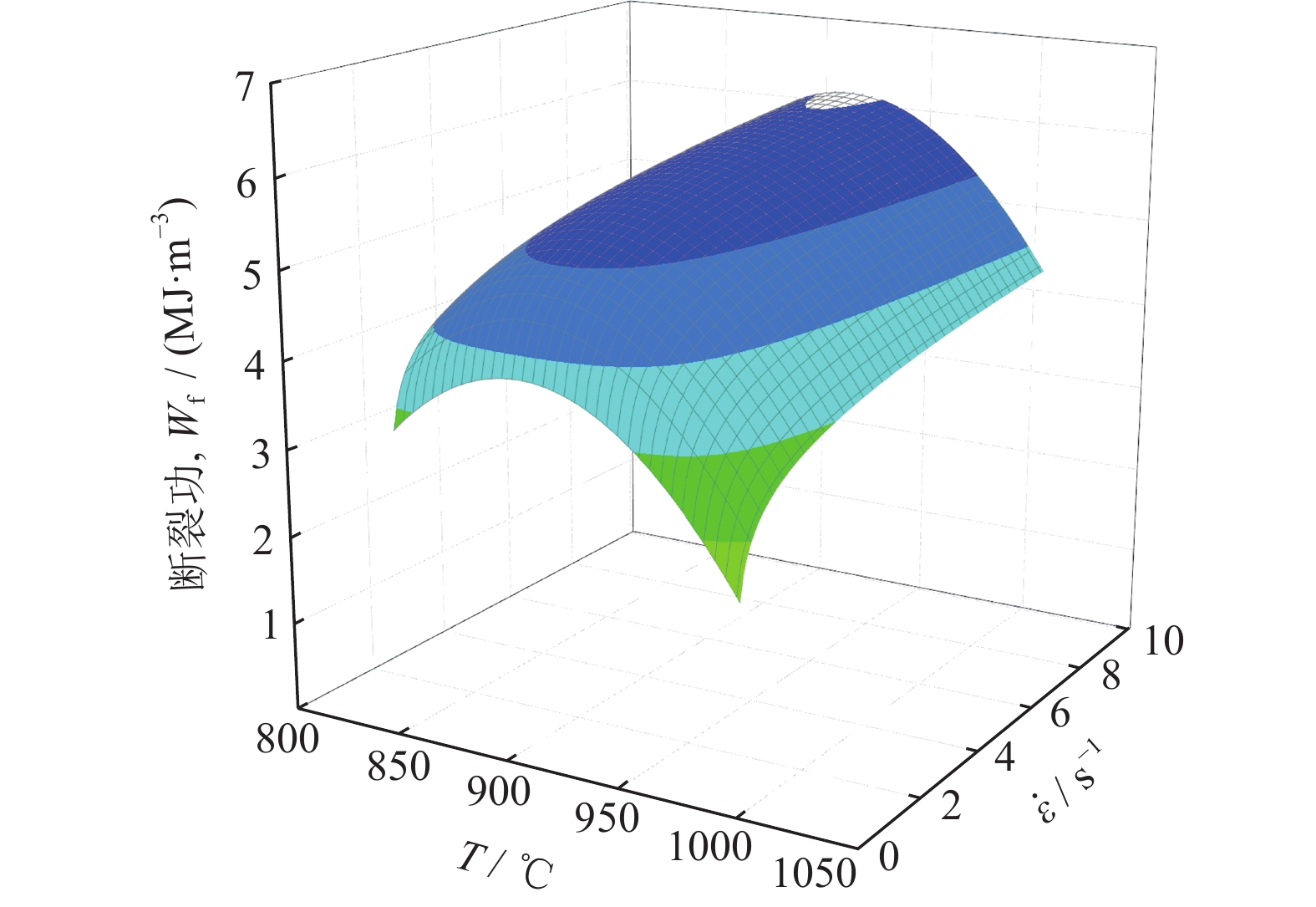
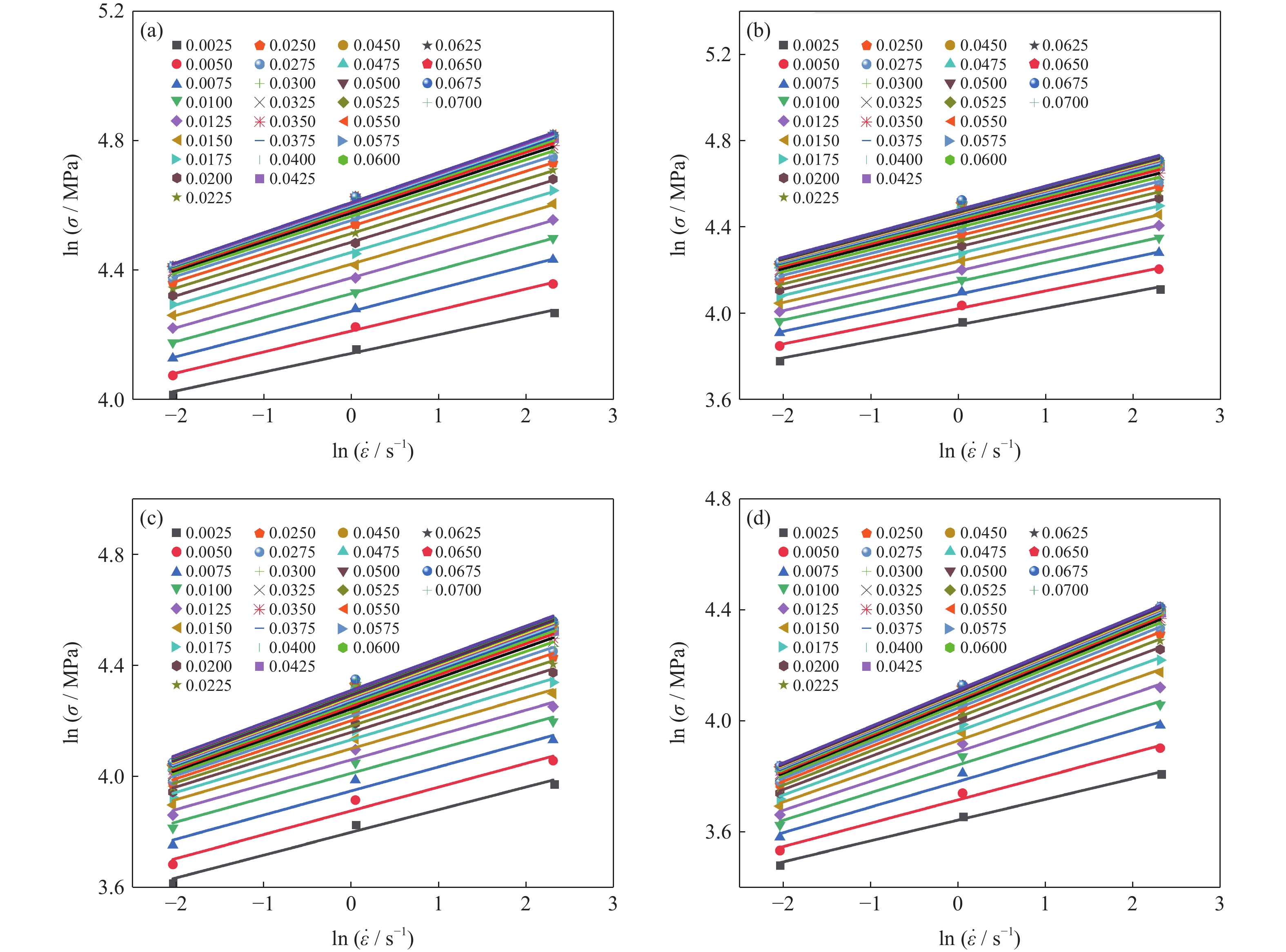
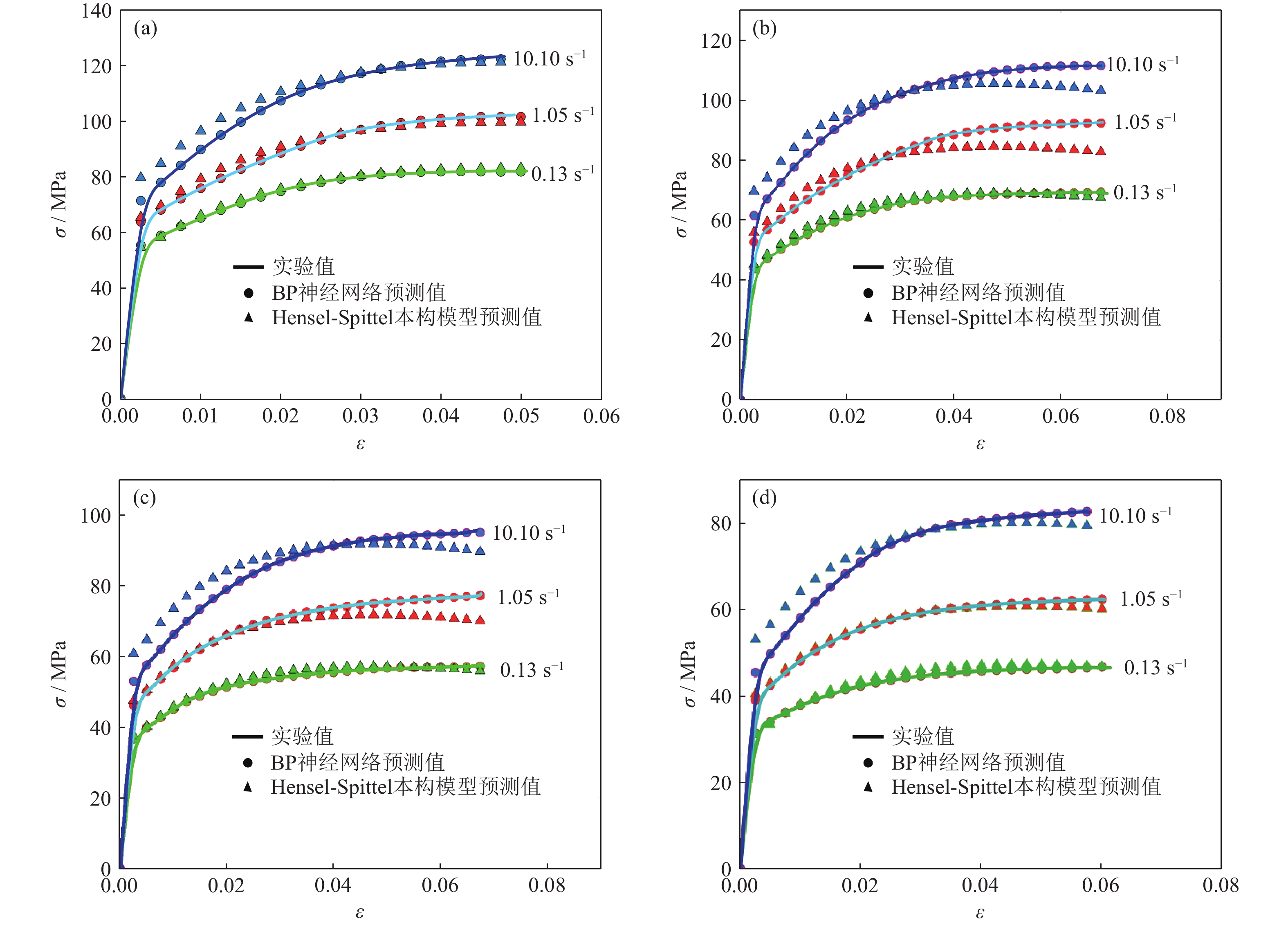
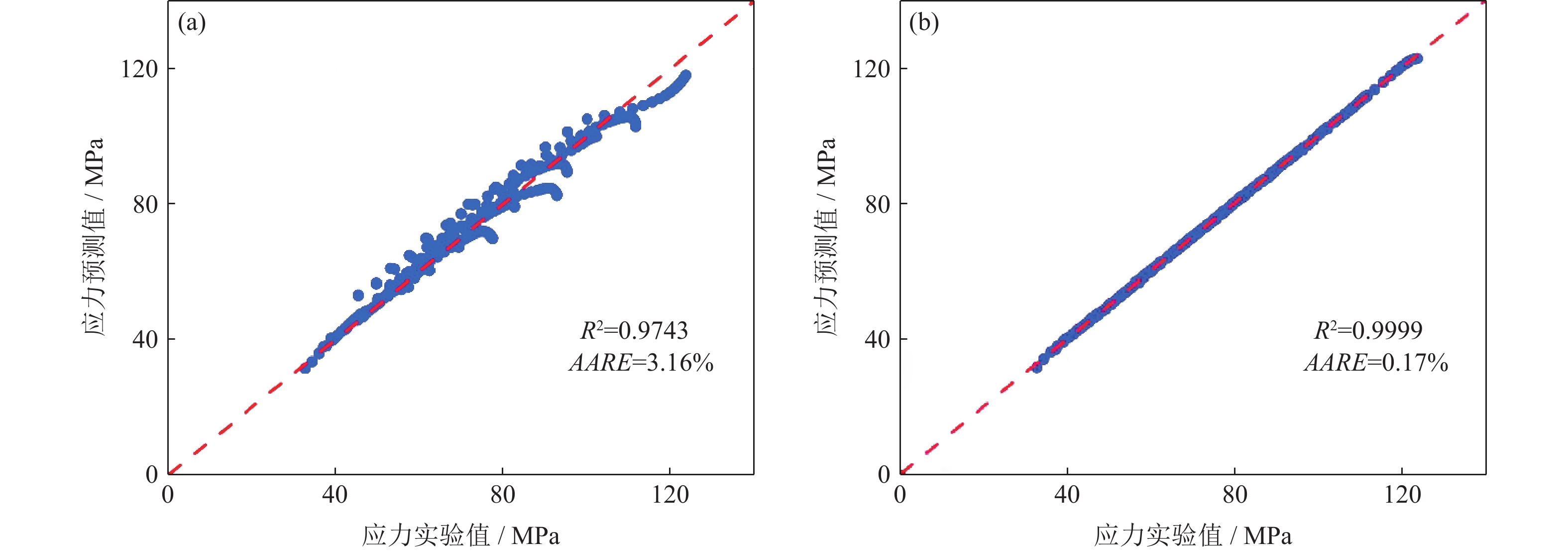
使用Gleeble-3500型热模拟机对粉末冶金Fe−2Cu−0.5C钢在变形温度850～1000 ℃、应变速率0.1～10.0 s−1下进行高温拉伸测试，定量分析变形温度、应变速率对粉末冶金钢高温拉伸变形行为的影响。通过应力应变曲线计算了该粉末冶金钢高温拉伸过程的断裂功，建立了断裂功与变形温度、应变速率的数学模型。基于Hensel-Spittel模型和BP神经网络模型建立了该粉末冶金钢的本构模型，用于表征其高温拉伸流变行为，并将两种模型的预测结果进行比较。结果表明：所建立的断裂功模型能够描述该粉末冶金钢在不同变形温度和应变速率下的抗断裂能力。Hensel-Spittel本构模型的预测值与实验值的平均绝对相对误差为3.16%，决定系数为0.9743，而BP神经网络模型的预测值与实验值的平均绝对相对误差为0.17%，决定系数为0.9999，说明BP神经网络模型的预测能力更强，能更好地表征粉末冶金Fe−2Cu−0.5C钢的高温拉伸流变行为。
Abstract:The elevated temperature tensile tests of the powder metallurgy (P/M) Fe−2Cu−0.5C steels at the deformation temperatures of 850~1000 ℃ and strain rates of 0.1~10.0 s−1 were carried out by Gleeble-3500 thermal simulator. The effects of deformation temperature and strain rate on the elevated temperature tensile deformation behaviors of the P/M steels were quantitatively analyzed. The fracture work of the P/M steels during the elevated temperature tensile was calculated using the stress-strain curves. The mathematical model characterizing the relationship of fracture work, deformation temperature, and strain rate was established. The Hensel-Spittel model and BP neural network model were used to establish the constitutive equation of the P/M steels to characterize the flow behaviors during the elevated temperature tensile. The predicted results of the two models were compared. The results show that the fracture work model can describe the capacity of the P/M steels to resist fracture at different deformation temperatures and strain rates. The average absolute relative error between the predicted values of Hensel-Spittel constitutive model and the experimental values is 3.16%, and the coefficient of determination is 0.9743. While the average absolute relative error and the coefficient of determination between the predicted values of BP neural network model and the experimental values are 0.17% and 0.9999, respectively, indicating that the BP neural network model has the stronger predictive capacity and can characterize the elevated temperature tensile deformation behaviors of the P/M Fe−2Cu−0.5C steels better.
-
对于一些价格昂贵、加工工艺复杂、加工周期长的不锈钢工件来说，修复再制造它们的受损疲劳破坏件无疑是一项重大的绿色工程，也是当代工业实现绿色生产的迫切需求。激光熔覆过程是一个变化较大的化学物理过程，熔覆结果主要受两方面的制约，首先是熔覆材料与基体界面间原子的相互扩散比较充分,形成稳定结合，其次是激光熔覆过程的工艺参数对熔覆质量至关重要。近年来，国内外学者对不锈钢激光熔覆进行了大量实验探索。王围等[1]以不锈钢液压油缸杆件为研究对象，采用熔覆技术对其再制造修复过程中的问题进行分析，说明了选用不锈钢粉末修复各种类型的液压油缸杆件在经济上和技术上的可行性。杨宁等[2‒7]基于激光熔覆不锈钢工艺参数与熔覆层质量的关系总结了扫描速度对于稀释率和熔覆层成型厚度的影响。作为一种表面修复工艺，激光熔覆的参数设定会对熔覆结果有重要影响，熔覆粉末的选用也决定着熔覆层结果的优劣。闫世兴和董世运[8]以不锈钢为基体，对激光熔覆Fe314合金粉末进行研究，结果表明其力学性能及熔覆层质量良好。张骁丽等[9]采用铝合金粉末选择性对零件部位进行激光熔化并优化熔覆过程工艺参数。蒲亚博[10]以3Cr14不锈钢为基体，对0.3C‒18Cr合金粉末进行研究，并通过优化工艺参数显著提高了熔覆层的硬度。冯嘉宁[11]在304奥氏体不锈钢表面制备Ni60合金涂层，大大提高了材料的耐磨性。朱国斌[12]在304不锈钢表面激光熔覆Ni基合金粉末，获得耐蚀性较好的涂层。杨丹等[13]研究了不同工艺参数对304不锈钢表面激光熔覆Ni基合金后熔覆层微观组织及硬度、耐磨、耐蚀性能的影响，并寻求最佳激光工艺参数，以期获得冶金结合较好，耐磨、耐蚀性能良好的熔覆层。郭泽东[14]采用矿用液压支柱的不锈钢材料作为基体，通过激光熔覆相应不锈钢粉末显著提高了它的硬度、耐磨性和耐腐性。Pinkerton和Li[15]对激光在不锈钢熔覆方面的应用作了研究，发现了主要输入工艺参数与成型试样的尺寸、特性以及表面粗糙度之间的联系。
镍基合金具有耐蚀、耐磨、抗高温蠕变和抗热氧化性的特点，是激光熔覆合金体系的主要材料之一[16]。为推动激光熔覆镍基合金粉末在特定不锈钢工件强化和修复上的应用，本文利用功率可调制碟片激光器在不同激光功率、扫描速度、送粉速度和光斑大小下对In625镍基合金粉末的激光熔覆进行研究，探索出了In625不锈钢镍基合金粉末在ZG06Cr19Ni4Mo不锈钢基板上的最优激光熔覆工艺参数，并总结了激光熔覆工艺参数对熔覆层高度、表面形态的影响规律。
1. 实验材料及方法
1.1 材料及设备
选用三维激光加工系统来进行激光熔覆实验，激光熔覆系统及加工原理如图1所示。整个系统包括碟片式激光器（德国TRUMPF TruDisk碟片式激光器，激光光束波长为1020 nm，光束质量≥2 mm·mrad，功率≤2000 W）、六自由度的酷卡六轴工业机器人运动系统、激光熔覆系统（包括激光焊接头、气流式双筒同步式GTV送粉器）、稀有气体保护系统（保护气体为氮气、送粉气体为氦气）、工装夹具系统、机器人控制柜和冷水机等。
选用ZG06Cr13Ni4Mo不锈钢板作为基材，基体尺寸为200 mm×200 mm×12 mm，熔覆材料选择In625镍基合金粉末，粒度为50～150目，在此粒度范围内，激光熔覆效果受粒度影响不明显。表1所示为熔覆粉末与不锈钢基体的化学成分。
表 1 In625合金粉与ZG06Cr13Ni4Mo不锈钢的化学成分（质量分数）Table 1. Chemical composition of the In625 alloy powders and ZG06Cr13Ni4Mo steels% 材料 C Si Mn Nb，Ta Al P S Cr Mo Ni Ti Cu Fe 不锈钢 ≤0.06 ≤1.00 ≤1.00 — — ≤0.030 ≤0.030 11.5～14.0 0.4～1.0 3.5～4.5 — — 余量 In625 ≤0.10 ≤0.50 ≤0.50 3.15～4.15 ≤0.40 ≤0.015 ≤0.015 20.0～23.0 8.0～10.0 ≥58.0 ≤0.4 ≤0.5 ≤5.0 1.2 实验准备及参数设置
在进行激光熔覆前，要对选择的熔覆粉末进行加热除湿，将足量In625合金粉在干燥箱中以90 ℃恒温干燥处理2 h。利用打磨机对不锈钢板实验面进行磨光处理，使用工业乙醇浸湿的无尘纸擦拭、清洗、去除不锈钢板表面的杂质与油污。
根据企业实际生产经验，通过控制激光焊接头到基板的距离来调节光斑大小为4 mm，搭接率为50%（步进2 mm），按实际工程需要，将熔覆厚度控制在1.0～1.2 mm范围内，故送粉速度控制在2～3 r·min−1（60～90 g·min−1），同时分别选用氦气、氮气作为送粉气与保护气，其流量设定分别为4.7和12.0 L·min−1。在遵循保证实验效果且减少实验次数的原则下，本次实验主要控制激光功率和扫描速度这两个变量，分别用A和B来进行表示。激光功率（A）控制在1500～2000 W之间，扫描速度（B）的变化范围为3.3～6.7 mm·s−1，采用响应曲面法拟定双因素3水平的实验方案。
响应面分析法也称作响应曲面设计方法，是一种多元线性回归分析方法。通过确定实验指标及因素水平编码，结合相应正交表对实验进行编号，然后依据编号进行实验并获得相应数据，并应用回归方程对因子与目标值之间的数学关系进行拟合，基于回归方程确定最佳参数。激光熔覆工艺参数与编码水平见表2，实验方案如表3所示。
表 2 激光熔覆工艺参数与编码水平Table 2. Laser cladding process parameters and coding level编码水平 激光功率 / W 扫描速度 / (mm·s−1) −1 1500 3.3 0 1750 5.0 1 2000 6.7 表 3 实验方案Table 3. Designed experiment实验编号 激光功率 / W 扫描速度 / (mm·s−1) 1 1500 3.3 2 1500 5.0 3 1500 6.7 4 1750 3.3 5 1750 5.0 6 1750 6.7 7 2000 3.3 8 2000 5.0 9 2000 6.7 10 1750 5.0 11 1750 5.0 12 1750 5.0 13 1750 5.0 2. 结果与分析
2.1 方差分析
按照上述设计的实验方案以及设置的工艺参数进行激光熔覆实验，其中4组重复实验结果用于获取激光功率与扫描速度对熔覆层高度交互影响的响应面，剩余9组实验的多道搭接熔覆区宏观形貌如图2所示。由图可知，按照从左到右（扫描速度为单一变量）、从上到下（激光功率为单一变量）的顺序依次给熔覆区域进行排序，分别为区域1～9，各个区域均为边长为40 mm的方形，9个区域基本都能够完整成形，涂层表面未出现明显的裂纹、塌陷等缺陷。熔覆层高度测量结果如表4所示。
![]()
图 2 不同工艺参数下的熔覆层外观形貌Figure 2. Appearance morphology of the cladding layer operated by the different process parameters表 4 熔覆层高度测量结果Table 4. Measurment results of the cladding layer height区域编号 1 2 3 4 5 6 7 8 9 高度 / mm 1.3～1.5 1.2～1.4 0.9～1.0 1.3～1.4 1.1～1.2 0.6～0.8 1.2～1.3 1.0～1.1 0.4～0.6 为了得到熔覆层高度（H）的回归模型，利用Desigh-Expert软件中的Response Surface模块对数据进行函数拟合，并采用方差分析方法对模型进行评估。表5是由响应面分析软件直接导出的具体方差分析结果，其中均方值（Mean square）表征影响因子对于响应的影响程度，数值越大越显著；P值是方差分析里面对模型和模型系数进行显著性检验得到的结果，表示分析对象的显著性，若显著，则说明该模型是有效的；失拟项（lack of fit）是评判拟合方程可靠度的重要目标，若失拟项结果不显著则说明拟合方程可靠性强。
表 5 熔覆层高度的方差分析Table 5. Variance analysis for the cladding layer height变异来源 平方和 自由度 均方值 F值 P值 显著性 模型 0.9200 5 0.1800 46.280 ＜0.0001 S A 0.1800 1 0.1800 46.040 0.0003 — B 0.6700 1 0.6700 167.000 ＜0.0001 — AB 0.0160 1 0.0160 3.910 0.0884 — A2 0.0001 1 0.0001 0.025 0.8790 — B2 0.0470 1 0.0470 11.870 0.0108 — 残差 0.0280 7 0.0040 — — — 失拟项 0.0220 3 0.0073 4.900 0.0795 NS 纯误差 0.0060 4 0.0015 — — — 注：S=Significant，NS=Not Significant 表5的熔覆层高度方差分析结果表明，在送粉量一定的情况下，扫描速度（B）对于熔覆层高度的影响十分显著（P＜0.0001），对激光功率（A）、AB、B2的影响比较显著（P＜0.1000），对A2的影响不显著（P＞0.1000）。通过对比均方值大小可知，影响要素的重要程度为B＞A。失拟项的P值表现为不显著，说明关于熔覆层高度的回归方程有良好的可靠性。
采用Desigh-Expert中的摄动图和响应面来评估各个工艺参数对熔覆层高度的影响，结果如图3所示，其中纵坐标表示熔覆层高度，横坐标表示工艺参数对熔覆层高度的影响系数（a）。由图3可知，在预定范围内，熔覆层厚度随着扫描速度（B）的增加而逐渐递减，这是由于激光对同步输送的粉末熔融时间减少，热输入不足，可熔覆的有效粉末量减少；激光功率（A）与熔覆层高度呈近似线性关系（系数为负），这是因为稀释率的高低与激光功率的变动密切相关，基体单位面积上获取的能量跟激光功率的加大明显上升，熔池的深度也明显增大，且其变化趋势要远大于熔覆层高度的变化。
![]()
图 3 工艺参数影响熔覆层高度的摄动图Figure 3. Effect of the process parameters on the cladding layer height in perturbation diagram利用Design-Expert软件得到回归预测模型和方差分析，其中激光功率（A）和扫描速度（B）的相互影响模型如图4所示。由图中激光功率和扫描速度对于熔覆层厚度的交互影响（AB）响应面可以看出，响应值（H）显然由于因素A与因素B的降低而加大。这也说明了，在保证送粉速度不变时，较低的激光功率与小的扫描速度相结合能够形成高的熔覆层。
![]()
图 4 激光功率和扫描速度对于熔覆层厚度交互影响的响应面Figure 4. Response surface of the laser power and scanning speed to the interactive influence of the cladding layer height2.2 金相组织
为了进一步研究各个激光熔覆参数下熔覆涂层金相组织的变化，对各个成形区域进行切割取样，观察熔覆层横截面的金相组织。按照试样选取、镶嵌、试样粗磨、试样精磨、试样抛光、试样腐蚀的金相检测步骤对试样进行前期处理，侵蚀过程采用液体体积比V（FeCl3）:V（HCl）:V（H2O）=1:10:20的腐蚀液进行1 min的化学腐蚀。待试样充分腐蚀后，使用GX50A倒置金相显微镜观察熔覆层垂直于扫描方向的横截面的几何形貌及金相组织，结果如图5所示。
![]()
图 5 不同工艺参数下的熔覆层横截面金相组织：（a）1500 W，3.3 mm/s；（b）1500 W，5.0 mm/s；（c）1500 W，6.7 mm/s；（d）1750 W，3.3 mm/s；（e）1750 W，5.0 mm/s；（f）1750 W，6.7 mm/s；（g）2000 W，3.3 mm/s；（h）2000 W，5.0 mm/s；（i）2000 W，6.7 mm/sFigure 5. Metallographic structure of the cladding layer in the cross section under the different process parameters: (a) 1500 W, 3.3 mm/s; (b) 1500 W, 5.0 mm/s; (c) 1500 W, 6.7 mm/s; (d) 1750 W, 3.3 mm/s; (e) 1750 W, 5.0 mm/s; (f) 1750 W, 6.7 mm/s; (g) 2000 W, 3.3 mm/s; (h) 2000 W, 5.0 mm/s; (i) 2000 W, 6.7 mm/s从图5可以看出，技术设定值的不同很大程度上影响着金相组织，图5（a）、图5（b）、图5（c）的金相组织显示熔覆区与基体区的结合层不明显，原因是激光功率偏小，熔覆金属粉和基体摄入的能量不足，无法实现很好的熔融，很难形成明显的结合层。从图5（d）、图5（e）、图5（f）可以看出，随着激光功率的增加，熔覆区和熔化区之间开始形成比较明显的结合层，但是由于激光功率还偏小，图5（d）中存在少量裂纹，图5（e）中存在气孔，图5（f）中有较多裂纹和气孔，原因是在激光功率一定时，扫描速率加快会使热量输入有所下降，组织中出现气孔、裂纹等缺陷也在逐渐增多；在2000 W激光功率下得到的金相组织质量明显提高，在图5（g）、图5（h）、图5（i）中没有发现明显的气孔、裂纹存在，主要区别是结合层的厚度由于扫描速度的加快而有所降低。结合实际生产要求以及产品对各个技术设定值的响应程度，最终确定最优的工艺参数为激光功率2000 W, 扫描速度5 mm·s−1。
2.3 最优工艺参数熔覆实验
采用前期实验获得的技术设定值重新安排实验，并对实验后的熔覆层样品进行相应的硬度检测，结果如下表6。基体材料ZG06Cr13Ni4Mo的布氏硬度为HB 217～HB 286，硬度实验的数据证实，合金熔覆层与所选不锈钢的硬度值十分吻合。将磨制好的试样置于70 ℃的体积比1:1盐酸水溶液中进行侵蚀，在低倍显微镜下观察熔覆层低倍组织形貌，结果如表7和图6所示。图中基材是ZG06Cr13Ni4Mo不锈钢，熔覆材料是In625合金粉，熔覆层截面无明显裂纹、气孔。综合所述，采用优化后的工艺参数激光熔覆In625合金粉能够获得质量良好的熔覆层以及合格的产品。
表 6 硬度实验结果Table 6. Hardness test results硬度，HB5/750 平均硬度，HB5/750 277 279 278 278 注：HB5/750代表用直径5 mm的硬质合金球在7.355 kN试验力下保持10～15 s测定的布氏硬度值 表 7 样品缺陷列表Table 7. List of cdefects缺陷类型 裂纹 未熔合 未焊透 气孔 夹渣 评价 无 无 无 无 无 3. 结论
（1）在ZG06Cr13Ni4Mo不锈钢基体上熔覆In625合金粉，在维持送粉量不变的条件下，熔覆层高度对激光功率与扫描速度的响应都比较明显。激光功率和扫描速度直接决定着熔覆层能否完整形成，熔覆层的形成由单位时间、单位面积的激光能量决定，激光功率和扫描速度的大小决定输入能量的强弱。
（2）要获得优质的熔覆层，激光功率与扫描速度均存在一个合理的限度；维持扫描速度等参数稳定的同时，提高激光功率，合金涂层质量明显提升。
（3）在考虑再制造时间成本和实际加工要求的情况下，熔覆工艺的最佳参数组合为激光功率2000 W，送粉量84 g·min−1，扫描速度5 mm·s−1，在此参数下获得的熔覆试样具有高质量的熔覆层，无气孔和裂纹，且表面光滑，其显微硬度与基材自身硬度一致。
-
![]()
图 2 断裂功模型预测值与实验测算值
Figure 2. Fracture work values of the model prediction and the experimental calculation
![]()
图 3 断裂功与变形温度及应变速率之间的关系
Figure 3. Relationships of the fracture work, deformation temperature, and strain rate
![]()
图 4 不同变形温度下lnσ−ln
$ \dot \varepsilon $ 关系曲线：（a）850 ℃；（b）900 ℃；（c）950 ℃；（d）1000 ℃Figure 4. Relationship of lnσ−ln
$ \dot \varepsilon $ at the different deformation temperatures: (a) 850 ℃; (b) 900 ℃; (c) 950 ℃; (d) 1000 ℃![]()
图 5 不同应变下m3+m7T与温度关系曲线
Figure 5. Relationship between m3+m7T and temperature under the different strains
![]()
图 7 不同应变速率下S与ln(ε+1)关系曲线
Figure 7. Relationship between S and ln(ε+1) at the different strain rates
![]()
图 8 不同变形温度下lnσ与ε关系：（a）850 ℃；（b）900 ℃；（c）950 ℃；（d）1000 ℃
Figure 8. Relationship of lnσ and ε at the different deformation temperatures: (a) 850 ℃; (b) 900 ℃; (c) 950 ℃; (d) 1000 ℃
![]()
图 11 不同温度下两种模型的应力预测值与实验值：（a）850 ℃；（b）900 ℃；（c）950 ℃；（d）1000 ℃
Figure 11. Experimental stress and the predicted stress by Hensel-Spittel and BP modes at the different temperatures: (a) 850 ℃; (b) 900 ℃; (c) 950 ℃; (d) 1000 ℃
-
[1] 冯士超, 王艳红, 丁瑞锋. 粉末冶金在钢铁企业的应用及发展前景. 粉末冶金技术, 2015, 33(4): 296 DOI: 10.3969/j.issn.1001-3784.2015.04.011 Feng S C, Wang Y H, Ding R F. Application and prospect of powder metallurgy in steel enterprises. Powder Metall Technol, 2015, 33(4): 296 DOI: 10.3969/j.issn.1001-3784.2015.04.011
[2] Tan Z Q, Zhang Q, Guo X Y, et al. New development of powder metallurgy in automotive industry. J Cent South Univ, 2020, 27(6): 1611 DOI: 10.1007/s11771-020-4394-y
[3] Vasanthakumar P, Sekar K, Venkatesh K. Recent developments in powder metallurgy based aluminium alloy composite for aerospace applications. Mater Today, 2019, 18(7): 5400
[4] Shang F, Zhou H X, Qiao B, et al. Application of metal powder metallurgy technology in preparation of friction materials of the railway vehicles. Adv Mater Res, 2011, 1334(287-290): 2987
[5] 包崇玺, 曹阳, 易健宏, 等. 高密度铁基粉末冶金零件制备技术. 粉末冶金技术, 2022, 40(5): 458 Bao C X, Cao Y, Yi J H. et al. Preparation processes of high density iron-based powder metallurgy parts. Powder Metall Technol, 2011, 2022, 40(5): 458
[6] Guo B, Li Q, Wu H, et al. Influences of impact energy on the densification and mechanical properties of powder metallurgical Fe−C−Cu preforms during a powder-forged process. J Mater Res Technol, 2020, 9(6): 13914 DOI: 10.1016/j.jmrt.2020.09.084
[7] Rodriguez A K, Ayoub G A, Mansoor B, et al. Effect of strain rate and temperature on fracture of magnesium alloy AZ31B. Acta Mater, 2016, 112: 194 DOI: 10.1016/j.actamat.2016.03.061
[8] Guo Y, Zhou M X, Sun X D, et al. Effects of temperature and strain rate on the fracture behaviors of an Al−Zn−Mg−Cu alloy. Materials, 2018, 11(7): 1233 DOI: 10.3390/ma11071233
[9] He A, Xie G L, Zhang H L, et al. A modified Zerilli-Armstrong constitutive model to predict hot deformation behavior of 20CrMo alloy steel. Mater Des, 2014, 56: 122 DOI: 10.1016/j.matdes.2013.10.080
[10] Song B, Sanborn B. A modified Johnson-Cook model for dynamic response of metals with an explicit strain- and strain-rate-dependent adiabatic thermosoftening effect. J Dyn Behav Mater, 2019, 5(3): 212 DOI: 10.1007/s40870-019-00203-0
[11] 尹小燕, 骆静, 朱杰. 基于Hansel-Spittel模型的齿环用HAl61-4-3-1合金本构模型构建. 重庆理工大学学报(自然科学), 2021, 35(1): 111 Yin X Y, Luo J, Zhu J. Construction of high-temperature constitutive model of HAl61-4-3-1 alloy for synchronizer ring based on Hansel-Spittel mode. J Chongqing Univ Technol Nat Sci, 2021, 35(1): 111
[12] Meng B, Wan M, Wu X D, et al. Constitutive modeling for high-temperature tensile deformation behavior of pure molybdenum considering strain effects. Int J Refract Met Hard Mater, 2014, 45: 41 DOI: 10.1016/j.ijrmhm.2014.03.005
[13] Zhu H F, Liu J, Wu Y, et al. Hot deformation behavior and workability of in situ TiB2/7050Al composites fabricated by powder metallurgy. Materials, 2020, 13(23): 5319 DOI: 10.3390/ma13235319
[14] Zyguła K, Wojtaszek M, Śleboda T, et al. The analysis of hot deformation behavior of powder metallurgy Ti−10V−2Fe−3Al alloy using activation energy and Zener-Hollomon parameter. Procedia Manuf, 2020, 50: 546 DOI: 10.1016/j.promfg.2020.08.098
[15] Qu F S, Dong X F, Liu J Y, et al. The research on vacuum isothermal bloom for powder metallurgy V−5Cr−5Ti alloy guiding by the constitutive models and the error analysis. Int J Refract Met Hard Mater, 2018, 72: 349 DOI: 10.1016/j.ijrmhm.2018.01.007
[16] Wang J, Zhao G Q, Chen L, et al. A comparative study of several constitutive models for powder metallurgy tungsten at elevated temperature. Mater Des, 2016, 90: 91 DOI: 10.1016/j.matdes.2015.10.114
[17] 李强, 郭彪, 吴辉, 等. Fe−2Cu−0.5C粉末烧结钢高温拉伸流变应力及预测. 锻压技术, 2020, 45(8): 195 Li Q, Guo B, Wu H, et al. Flow stress and prediction on powder sintered steel Fe−2Cu−0.5C during high temperature tensile. Forg Stamp Technol, 2020, 45(8): 195
[18] Wu H, Wen S P, Huang H, et al. Hot deformation behavior and constitutive equation of a new type Al−Zn−Mg−Er−Zr alloy during isothermal compression. Mater Sci Eng A, 2016, 651: 415 DOI: 10.1016/j.msea.2015.10.122
[19] Wang H R, Wang W, Zhai R X, et al. Constitutive equations for describing the warm and hot deformation behavior of 20Cr2Ni4A alloy steel. Metals, 2020, 10(9): 1169 DOI: 10.3390/met10091169
[20] Qiao L, Deng Y, Liao M, et al. Modelling and prediction of thermal deformation behaviors in a pearlitic steel. Mater Today Commun, 2020, 25: 101134 DOI: 10.1016/j.mtcomm.2020.101134
[21] He J, Xiao Y, Liu J, et al. Model for predicting ductile fracture of SA508-3 steel undergoing hot forming. Mater Sci Technol, 2014, 30(10): 1239 DOI: 10.1179/1743284713Y.0000000443
[22] Xu R R, Li M Q, Li H. Kinetic analysis and strain-compensated constitutive models of Ti−42.9Al−4.6Nb−2Cr during isothermal compression. Prog Nat Sci Mater Int, 2020, 30(2): 260
[23] Xiao X, Liu G Q, Hu B F, et al. A comparative study on Arrhenius-type constitutive equations and artificial neural network model to predict high-temperature deformation behaviour in 12Cr3WV steel. Comput Mater Sci, 2012, 62: 227 DOI: 10.1016/j.commatsci.2012.05.053
[24] 王天祥, 鲁世强, 王克鲁, 等. Ti60合金的流变应力行为及人工神经网络本构模型. 特种铸造及有色合金, 2020, 40(9): 1019 DOI: 10.15980/j.tzzz.2020.09.022 Wang T X, Lu S Q, Wang K L, et al. Flow stress behavior and constitutive model base on artificial neural network of Ti60 alloy. Spec Cast Nonferrous Alloys, 2020, 40(9): 1019 DOI: 10.15980/j.tzzz.2020.09.022
[25] 周峰, 王克鲁, 鲁世强, 等. Ti−22Al−24Nb−0.5Y合金流动行为及BP神经网络高温本构模型. 材料工程, 2019, 47(8): 141 Zhou F, Wang K L, Lu S Q, et al. Flow behavior and neural network high temperature constitutive model of Ti−22Al−24Nb−0.5Y alloy. J Mater Eng, 2019, 47(8): 141
[26] Murugesan M, Sajjad M, Jung D W. Microstructure evaluation and constitutive modeling of AISI-1045 steel for flow stress prediction under hot working conditions. Symmetry, 2020, 12(5): 782 DOI: 10.3390/sym12050782
-
期刊类型引用(6)
1. 赵子锐，段绪星，陈青，任维泽，林益文，裴泽宇. 镍基合金激光熔覆研究进展及其在反应堆的应用展望. 激光杂志. 2025(02): 1-9 . 
百度学术
2. 姚开礼，高丽. 激光编程技术在汽车模具淬火过程中的参数优化与控制研究. 模具制造. 2024(01): 92-95 . 百度学术
3. 范仲华. 电磁场辅助激光熔覆制备IN718涂层的组织及性能研究. 工程机械. 2024(03): 158-162+13 . 百度学术
4. 王进才，霍晓阳，李雷，樊贝贝，米国发. 激光增材再制造20G/Inconel625复合板工艺研究. 特种铸造及有色合金. 2022(01): 99-103 . 百度学术
5. 王贵. 镍基合金粉末热喷涂后收缩气孔的研究. 冶金与材料. 2022(04): 10-12 . 百度学术
6. 王进才，霍晓阳，李雷，樊贝贝，米国发. Inconel625及其去Mo和Alloy686粉末激光熔凝性能研究. 特种铸造及有色合金. 2022(10): 1256-1262 . 百度学术
其他类型引用(5)
下载:
计量
- 文章访问数: 2051
- HTML全文浏览量: 60
- PDF下载量: 62
- 被引次数: 11
