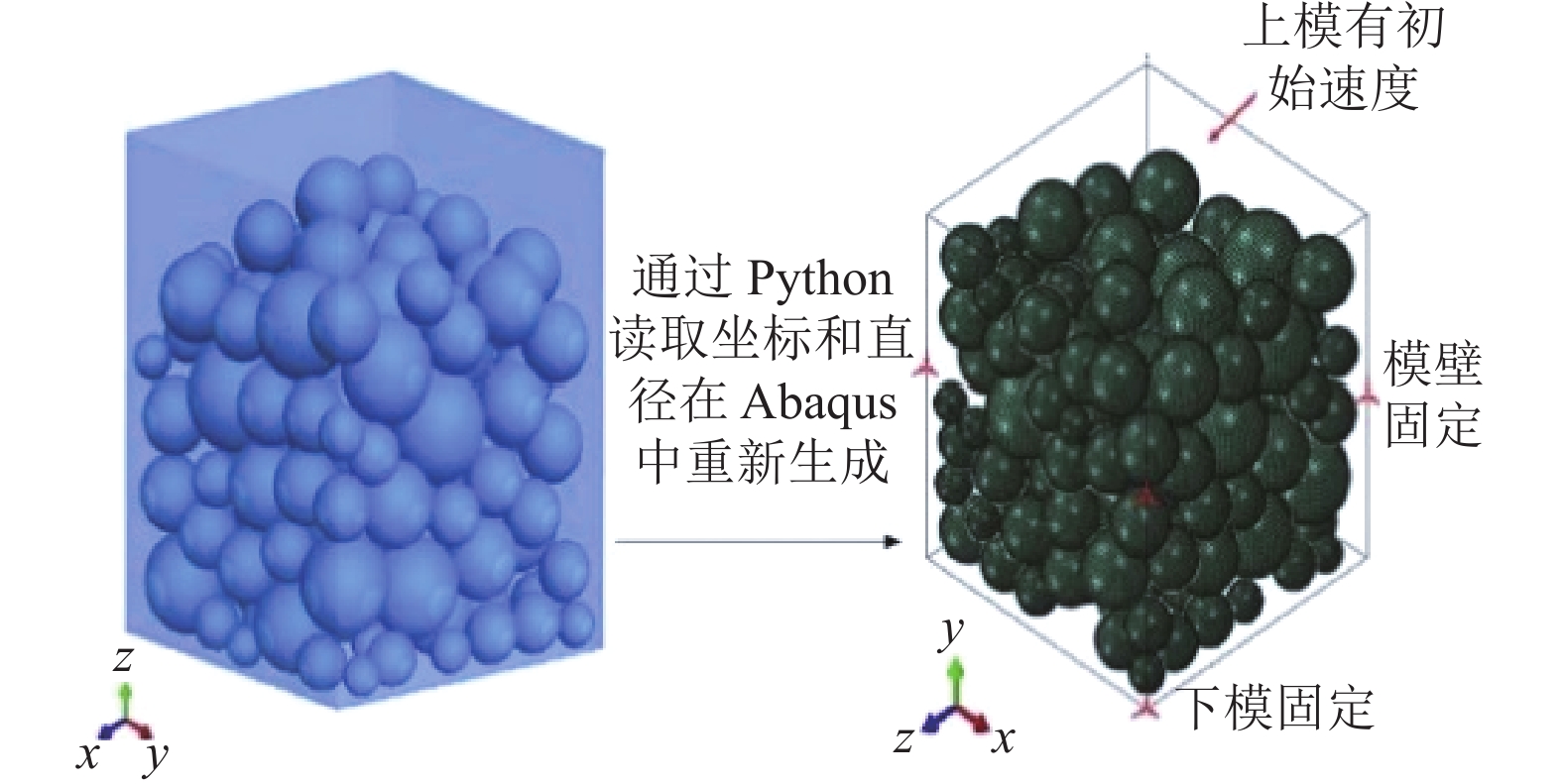
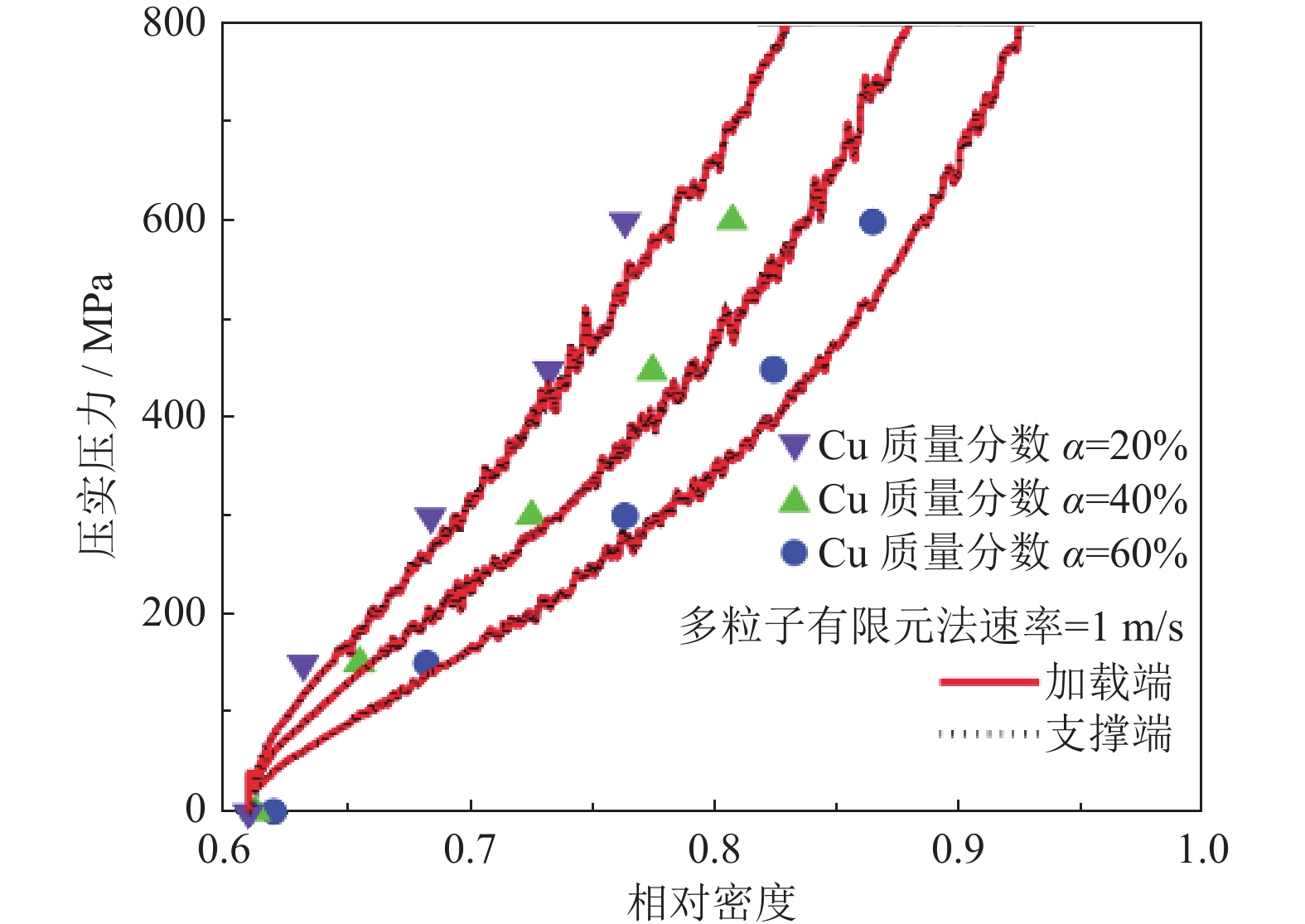
Application and development of numerical simulation on mesoscopic analysis of powder compaction
-
摘要:
数值模拟技术已经成为研究粉末压制过程的重要手段。研究人员运用离散单元法（discrete element method，DEM）从细观角度研究粉末颗粒的力学行为，分析力链特性及力链演化过程，揭示细观结构对宏观性质的影响；使用多粒子有限元法（multi-particle finite element method，MPFEM）从颗粒层面对不同粉末的压制变形机理进行研究。本文对离散单元法和多粒子有限元法两种数值模拟方法在粉末压制中的应用及发展进行综述，总结了多粒子有限元法在粉末压制数值模拟中的难点，分析得到在动态载作用下对粉末力链演化规律及颗粒致密机理的研究可作为未来探索方向的展望。
Abstract:In recent years, numerical simulation technology has become an important method to study the powder compaction process. The discrete element method (DEM) is used to study the mechanical behavior of powder particles from the mesoscopic perspective, analyze the characteristics and evolution process of force chain, and reveal the influence of the mesoscopic structure on the macroscopic properties. The multi-particle finite element method (MPFEM) is used to study the compression deformation mechanisms of the different powders at the particle level. The application and development of DEM and MPFEM on the powder compaction were reviewed in this paper, and the difficulties of MPFEM used in powder compaction were summarized.It was concluded that the study on the evolution law of powder force chain and the mechanism of particle densification under the dynamic loading could be regarded as a prospect for future exploration
-
氮化铝（AlN）陶瓷具有高导热性（理论可达320 W·m−1·K−1）、优异的绝缘性和耐高温性等优异性能，在军工、航天、电子信息、新能源等领域被广泛应用[1–4]。由于氮化铝晶体结构是高度共价键结合，纯氮化铝陶瓷必须在足够高的温度下才能烧结致密[5–7]。常见的烧结方法有反应烧结、常压烧结、热压烧结[8]、微波烧结[9−10]、激光烧结和放电等离子烧结[11−12]等。如图1所示，放电等离子烧结（spark plasma sintering，SPS）可以在短时间内将AlN粉末烧结成形，并且烧结试样具有很高的相对密度。放电等离子烧结是通过火花放电使得颗粒表面被活化，并通过颗粒之间的自加热使得粉末可以快速达到烧结温度，具有快速热传递和物质传递的特点[13]。纳米粉末具有较高的比表面积和表面能，烧结驱动力大、烧结活性高[14]，可以用来制备具有超细纳米晶的金属和非金属材料[15–17]。Wang等[18]利用放电等离子烧结制备单层碳纳米管增韧的AlN陶瓷。Shen等[19]以AlN陶瓷为基体，利用放电等离子烧结得到了原位生长的细长颗粒，提高了液相烧结硅铝氧氮复合材料的韧性。Basu等[20]采用相同方法获得了ZrO2–ZrB2复合材料。上述研究表明，采用放电等离子烧结工艺可以有效限制晶粒长大，实现材料快速致密化。但是，如果没有控制好烧结压力和烧结时间这两个因素，可能会导致材料微观结构不均匀，恶化材料性能。
本文选用纯纳米AlN粉和掺杂质量分数3%Y2O3的纳米AlN粉为原料，采用放电等离子烧结制备AlN陶瓷，研究烧结时间和烧结压力对AlN陶瓷相对密度、微观组织、力学性能和导热性能的影响，寻求纳米AlN粉末放电等离子烧结的最优工艺，制备出烧结致密、晶粒细小、性能优良的AlN陶瓷。
1. 实验材料与方法
实验所用纳米AlN粉末采用溶液燃烧合成法制备[21]，颗粒尺寸为80～100 nm，为减少原料粉末中的团聚，将其在无水乙醇中进行充分研磨，烘干、过筛。将纯纳米AlN粉命名为AY0，掺杂质量分数3%Y2O3的纳米AlN粉命名为AY3，分别进行放电等离子烧结，并对其烧结行为进行对比分析。具体实验流程如下：在直径为12.8 mm的石墨模具内部均匀铺入石墨纸，将1 g纳米AlN粉末装入模具中并用石墨纸封口，最后用石墨压头压实，石墨纸主要用来隔绝烧结样品与模具的接触，从而达到保护样品和模具的目的；将放好AlN粉末的石墨模具放于放电等离子烧结炉内，在＜10 Pa真空条件下，以100 ℃·min−1的升温速率从室温升到1500 ℃并烧结5～60 min，其中，烧结压力为40～60 MPa。
采用日本Rigaku公司D/MAX-RB12型X射线衍射仪（X-ray diffraction，XRD）分析样品物相。利用ZEISS ULTRA55场发射扫描电子显微镜（field emission scanning electron microscope，FESEM）观测试样断口形貌和晶粒大小。使用日本Auto Graph AGS-X万能试验机测试三点弯曲强度。通过阿基米德排水法原理测定试样密度。采用德国Netzsch LFA427激光导热分析仪测试导热性能。利用维氏硬度计（MH-6）测试试样硬度。所有样品经金刚石磨盘和抛光液研磨和抛光，再进行多次测试取平均值以减少误差，保障数据的准确性，其中样品热导和抗弯强度需测试3次以上，硬度测试需10次以上。
2. 结果与分析
2.1 烧结时间的影响
在放电等离子烧结过程中，脉冲电流能够在AlN颗粒间瞬时放电并升高温度，活化AlN颗粒表面并将颗粒烧结在一起。烧结时间影响晶界扩散时间，对AlN陶瓷粒径有重要影响，在恒温（1500 ℃）和恒压（40 MPa）下，选用不同烧结时间（5、30、60 min）进行放电等离子烧结。
表1为不同烧结时间放电等离子烧结AlN陶瓷的相对密度和晶粒尺寸。由于烧结时间为5 min时试样已经烧结致密，故将5 min视作初始烧结时间，此时的平均晶粒尺寸为初始晶粒尺寸。图2为不同烧结时间AY0和AY3试样断口的场发射扫描电子显微镜背散射图。由图可知，烧结时间从5 min增加至30 min，AY0试样的粒径大小从283 nm（图2（a））增加到332 nm（图2（b）），晶粒大小变化并不明显，此时烧结时间对晶粒大小的影响较小。当烧结时间进一步延长至60 min，晶粒发生明显长大，此时晶粒大小增至1.71 μm（图2（c）），是最初粒径的6倍。烧结时间充分会促进晶粒的迅速长大，并且长大速率逐渐加快。Pezzotti等[22]将添加质量分数5%Y2O3的微米AlN粉末烧结（1850 ℃、1 h）后循环热处理（1800 ℃、5 h和10 h），热处理5 h和10 h后试样的晶粒大小分别是原始烧结试样晶粒的1.2倍和1.5倍。可见在高温热处理时，晶粒长大速率远小于本实验中纳米粉末的晶粒长大速率。添加烧结助剂Y2O3后，烧结时间为5 min的AY3试样平均粒径达到767 nm（图2（d）），远大于同烧结时间无助剂烧结试样的晶粒尺寸。由于烧结助剂与氧化铝层结合，在晶界上形成第二相，加快了物质的迁移和扩散，从而加快了晶界的迁移速率，促进晶粒长大。烧结时间增加到30 min后，AY3试样平均晶粒尺寸增长至1.67 μm（图2（e）），这在初始晶粒尺寸的基础上仅长大了2倍左右。烧结时间加长到60 min后，AY3试样晶粒尺寸增至1.73 μm（图2（f）），晶粒长大变得非常缓慢。由此可知，添加烧结助剂Y2O3后，晶粒在短时间内迅速长大，继续延长烧结时间对于晶粒生长的促进作用不明显。
表 1 不同烧结时间放电等离子烧结试样的相对密度和平均晶粒尺寸Table 1. Relative densities and the average grain sizes of the SPS samples for the different sintering times样品 相对密度 / % 晶粒尺寸 / nm 5 min 30 min 60 min 5 min 30 min 60 min AY0 99.25 99.46 99.33 283 332 1710 AY3 99.12 99.39 99.07 767 1670 1730 ![]()
图 2 不同烧结时间AY0和AY3试样断口的场发射扫描电子显微镜背散射形貌：（a）AY0，5 min；（b）AY0，30 min；（c）AY0，60 min；（d）AY3，5 min；（e）AY3，30 min；（f）AY3；60 minFigure 2. FESEM back scatter images of the AY0 and AY3 fracture microstructures with the different sintering times: (a) AY0, 5 min; (b) AY0, 30 min; (c) AY0, 60 min; (d) AY3, 5 min; (e) AY3, 30 min; (f) AY3, 60 min对不同烧结时间制备的AY0和AY3试样进行力学性能测试，图3是试样硬度和抗弯强度随烧结时间变化情况。由图3（a）可知，随着烧结时间的增加，AY0和AY3试样的维氏硬度逐渐降低，这和晶粒大小的变化趋势是相关联的，即烧结时间延长后AlN晶粒长大，降低了其力学性能。AY0试样在烧结时间为5 min和30 min时，平均晶粒尺寸较为接近，因而其维氏硬度值也较为接近，分别为HV0.3 1479和HV0.3 1468，此时维氏硬度随烧结时间的增加降低程度较小。当烧结时间进一步增加到60 min后，晶粒已经长大到最初粒径尺寸的6倍左右，维氏硬度也出现了较大幅度的降低，此时维氏硬度为HV0.3 1414，维氏硬度的变化也符合Hall-Petch关系。AY3试样也表现出相似的变化趋势，烧结时间从5 min增长到30 min后，晶粒尺寸从767 nm长大到1.67 μm，维氏硬度值从HV0.3 1580降低到HV0.3 1501，其下降速率较大。当烧结时间增加到60 min后，AY3试样平均粒径从1.67 μm增长为1.73 μm，维氏硬度下降到HV0.3 1490。由于AY3试样晶粒尺寸在烧结时间进一步延长后长大不明显，其维氏硬度的降低程度也有限。从图3（b）可以看出，AY0和AY3试样抗弯强度的变化趋势基本和维氏硬度相似，也都符合Hall-Petch关系，晶粒尺寸越大，强度越低。
![]()
图 3 AY0和AY3试样硬度和抗弯强度与烧结时间关系：（a）维氏硬度；（b）抗弯强度Figure 3. Hardness and bending strength of AY0 and AY3 with the different sintering times: (a) Vickers hardness; (b) bending strength2.2 烧结压力的影响
由于实验所用的纳米AlN粉末采用溶液燃烧合成法制备，受溶液燃烧合成前驱体的微观结构影响，纳米AlN粉末中存在片状团聚体。在放电等离子烧结过程中对粉末进行压制，团聚体之间很容易形成拱桥效应。在烧结过程中，颗粒表面会快速到达高温并融化，并在粉末颗粒连接部位产生烧结颈。如果拱桥效应严重，会使得部分颗粒之间不能充分接触，形成烧结颈，颗粒间的物质传输也会受到影响。因此需要对粉末施加足够的压力来消除颗粒之间的拱桥效应，让粉末颗粒之间能够充分接触，促进烧结过程中颗粒的重排。此外，在烧结过程中施加一定的压力还能抑制晶粒的过分长大。针对放电等离子烧结过程中压力的作用很多学者展开了研究。Bernard-Granger等[23]通过对比放电等离子烧结与热压烧结，提出了“应力–速率”模型，研究了在高压力100 MPa时3Y-TZP纳米陶瓷的放电等离子烧结行为。结果表明，在烧结过程不同密度阶段和有效压力阶段，材料具有不同的密实化机理。Langer等[24−25]和Fang等[26]对比了亚微米氧化铝、钇稳定氧化锆和氧化锌的放电等离子烧结行为和热压烧结行为。研究认为，两种方法的致密化机理都是通过晶界扩散，相比于热压烧结，放电等离子烧结快速密实化主要是由于其在高温承受了更高的压力。
图4为不同烧结压力下放电等离子烧结制备AY0和AY3试样断口的场发射扫描电子显微形貌，表2为相应烧结试样的相对密度与平均晶粒尺寸。从图4可知，烧结压力从40 MPa升高到50 MPa时，试样平均粒径从283 nm（图4（a））减小到176 nm（图4（b）），且晶粒大小变得更加均匀，表明压力升高促进了颗粒的重排，使更多的颗粒相互接触，提供更多晶粒生长位点，有利于晶粒的细小均匀分布。压力继续从50 MPa升高到60 MPa时，AlN粒径反而增大到574 nm（图4（c）），且此时晶粒大小变得不均匀，出现晶粒尺寸为810 nm的异常长大晶粒。说明压力过大会造成小颗粒之间的合并。对比AY3试样断口形貌可以发现，不同压力下晶粒大小的变化趋势和AY0的一致，随着烧结压力的提升，晶粒尺寸在压力为50 MPa时达到最小，此时平均晶粒大小为190 nm（图4（e）），当烧结压力从50 MPa升高到60 MPa后，平均粒径大小从190 nm增长为353 nm（图4（f）），且没有出现异常长大的晶粒。这可能由于晶粒间第二相阻碍了晶粒之间的吞并与融合。烧结压力低于50 MPa时，添加烧结助剂有利于促进放电等离子烧结陶瓷的致密化和晶粒长大。
![]()
图 4 不同烧结压力下AY0和AY3试样断口场发射扫描电子显微镜背散射形貌：（a）AY0，40 MPa；（b）AY0，50 MPa；（c）AY0，60 MPa；（d）AY3，40 MPa；（e）AY3，50 MPa；（f）AY3；60 MPaFigure 4. FESEM back scatter images of the AY0 and AY3 fracture microstructures with the different pressures: (a) AY0, 40 MPa; (b) AY0, 50 MPa; (c) AY0, 60 MPa; (d) AY3, 40 MPa; (e) AY3, 50 MPa; (f) AY3, 60 MPa表 2 不同烧结压力下试样的平均晶粒尺寸和相对密度Table 2. Average grain sizes and the relative densities of the SPS samples under the different pressures样品 相对密度 / % 晶粒尺寸 / nm 40 MPa 50 MPa 60 MPa 40 MPa 50 MPa 60 MPa AY0 99.06 99.56 99.48 283 176 574 AY3 99.27 99.44 99.52 513 190 353 图5为AY0和AY3试样维氏硬度和抗弯强度随烧结压力的变化曲线。从图5（a）可知，当烧结压力为50 MPa时，AY0和AY3试样的维氏硬度最高分别达到HV0.3 1495和HV0.3 1585。烧结压力为40 MPa和60 MPa时，AY0和AY3试样的维氏硬度均发生降低。通过对比发现，维氏硬度的变化趋势和平均晶粒尺寸的变化趋势相吻合，即在压力为50 MPa时，AlN晶粒尺寸最小，力学性能最优，这也符合Hall-Petch关系。AY0试样在60 MPa时的维氏硬度为HV0.3 1434，低于压力为40 MPa时的维氏硬度HV0.3 1479，这是由于压力60 MPa时，试样晶粒异常长大，大尺寸晶粒降低了局部硬度。当烧结压力为50 MPa和60 MPa时，AY3试样维氏硬度均为HV0.3 1585，略高于40 MPa时的维氏硬度HV0.3 1580。尽管烧结压力为60 MPa时，其粒径要高于50 MPa时的晶粒尺寸，但硬度并未下降，这归因于在更高压力下部分缺陷（如气孔）会被消除，抵消了部分由于晶粒长大造成的力学性能下降。从图5（b）可以看出，当烧结压力为50 MPa时，AY0和AY3试样的抗弯强度最高分别达到473.25 MPa和386.52 MPa。烧结压力为40 MPa和60 MPa时，AY0和AY3试样的抗弯强度均发生降低，与维氏硬度变化趋势基本保持一致。
![]()
图 5 AY0和AY3试样力学性能随压力变化关系：（a）维氏硬度；（b）抗弯强度Figure 5. Mechanical properties of AY0 and AY3 under the different pressures: (a) Vickers hardness; (b) bending strength2.3 AlN陶瓷强度与热导率分析
图6为AY0和AY3试样的晶粒尺寸（d，μm）与抗弯强度关系曲线，两者变化趋势复合Hall-Petch关系。如图所示，在相同晶粒尺寸下，添加质量分数3%Y2O3的AlN陶瓷具有更高的抗弯强度。这归因于烧结助剂Y2O3的加入净化了晶界，提高了晶界的结合强度，阻止了裂纹在晶界上的扩展。
![]()
图 6 AY0和AY3试样晶粒尺寸与抗弯强度关系Figure 6. Relationship between the grain size and bending strength of the AY0 and AY3 samples在不同烧结时间下测定AY0和AY3试样热导率，结果如图7（a）所示，添加了Y2O3烧结助剂的试样热导率普遍较高。根据图7（b）X射线衍射分析结果可知，烧结后有钇铝酸盐相生成，这表明在烧结过程中，烧结助剂可以与氧结合生成钇铝酸盐，防止氧进入AlN晶格，避免更多的铝空位形成，引起声子散射，影响热导率。同时从图7（a）中可以看出，热导率随着烧结时间的延长而上升，在烧结时间为5～30 min时，AY0试样晶粒生长较慢，其热导率的上升速率较慢；在烧结时间为30～60 min时，晶粒长大迅速，热导率随烧结时间的延长上升较快。在烧结时间为5～30 min时，AY3试样晶粒生长较快，其热导率快速上升；在烧结时间为30～60 min时，晶粒长大速率变慢，其热导率的增长也变慢。烧结时间越长，晶粒尺寸越大，晶界数量相应减小，在热传导时对声子的散射也会减少。在烧结时间为60 min时，AY0和AY3试样热导率达到最大，分别为49.70 W·m−1·K−1和55.99 W·m−1·K−1。
![]()
图 7 AY0和AY3试样热导率（a）和物相组成（b）随烧结时间变化Figure 7. Thermal conductivity (a) and phases composition (b) of AY0 and AY3 with the different sintering times3. 结论
（1）以纯纳米AlN粉和掺杂质量分数3%Y2O3的纳米AlN粉为原料，在40～60 MPa下1500 ℃放电等离子烧结5～60 min，均可获得相对密度＞99%的AlN陶瓷。
（2）随着放电等离子烧结时间的延长，AlN陶瓷晶粒长大。当烧结时间从5 min延长至60 min时，AY0和AY3的平均晶粒尺寸分别增大至1.71 μm和1.73 μm，硬度分别降至HV0.3 1414和HV0.3 1490，弯曲强度分别降至297.56 MPa和370.46 MPa，导热性能分别增至49.70 W·m−1·K−1和55.99 W·m−1·K−1。
（3）放电等离子烧结压力增大能够有效细化AlN晶粒尺寸。当烧结压力为50 MPa时，平均晶粒尺寸最小，AY0和AY3的平均晶粒尺寸分别为176 nm和190 nm，硬度分别为HV0.3 1495和HV0.3 1585，抗弯强度分别为386.52 MPa和473.25 MPa，细化晶粒明显提高了AlN陶瓷的硬度和抗弯强度；继续增大压力，力学性能反而下降。
（4）在相同放电等离子烧结工艺下，添加烧结助剂Y2O3能够有效提升AlN陶瓷的综合性能。
-
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
-
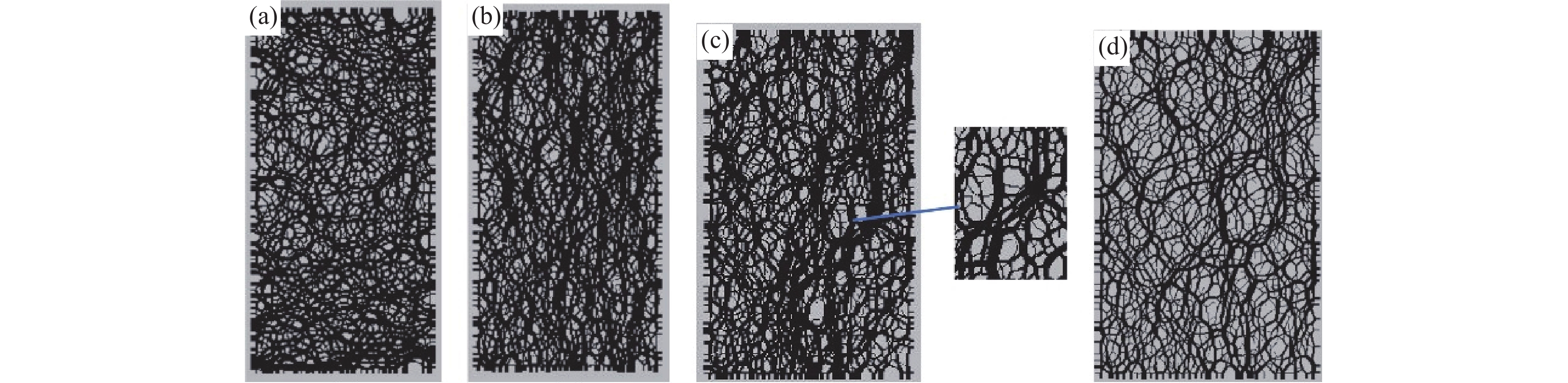
[1] 孙其诚, 刘晓星, 张国华, 等. 密集颗粒物质的介观结构. 力学进展, 2017, 47: 263 DOI: 10.6052/1000-0992-16-021 Sun Q C, Liu X X, Zhang G H, et al. The mesoscopic structures of dense granular materials. Adv Mech, 2017, 47: 263 DOI: 10.6052/1000-0992-16-021
[2] 尤萌萌, 潘诗琰, 申小平, 等. 粉末压制过程数值模拟的研究现状及展望. 粉末冶金工业, 2017, 27(4): 49 You M M, Pan S Y, Shen X P, et al. Current progress and prospect of numerical simulation in powder compaction. Powder Metall Ind, 2017, 27(4): 49
[3] 郭岩岩, 历长云, 冀国良, 等. 粉末致密化过程数值模拟研究现状. 材料导报, 2022, 36(18): 174 DOI: 10.11896/cldb.20080161 Guo Y Y, Li C Y, Ji G L, et al. Research status of numerical simulation of powder densification process. Mater Rep, 2022, 36(18): 174 DOI: 10.11896/cldb.20080161
[4] 孙其诚, 王光谦. 颗粒物质力学导论. 北京: 科学出版社, 2009 Sun Q C, Wang G Q. Introduction to the Mechanics of Particulate Matter. Beijing: Science Press, 2009
[5] 颜士伟, 黄尚宇, 胡建华, 等. 数值仿真技术在粉末冶金零件制造中的应用及研究进展. 粉末冶金技术, 2017, 35(1): 57 DOI: 10.3969/j.issn.1001-3784.2017.01.010 Yan S W, Huang S Y, Hu J H, et al. Development and application of numerical simulation in powder metallurgy manufacturing. Powder Metall Technol, 2017, 35(1): 57 DOI: 10.3969/j.issn.1001-3784.2017.01.010
[6] Sun Q C, Jin F, Liu J G. Understanding force chains in dense granular materials. Int J Mod Phys B, 2010, 24(29): 5743 DOI: 10.1142/S0217979210055780
[7] Guo P J. Critical length of force chains and shear band thickness in dense granular materials. Acta Geotech, 2012, 7: 41 DOI: 10.1007/s11440-011-0154-3
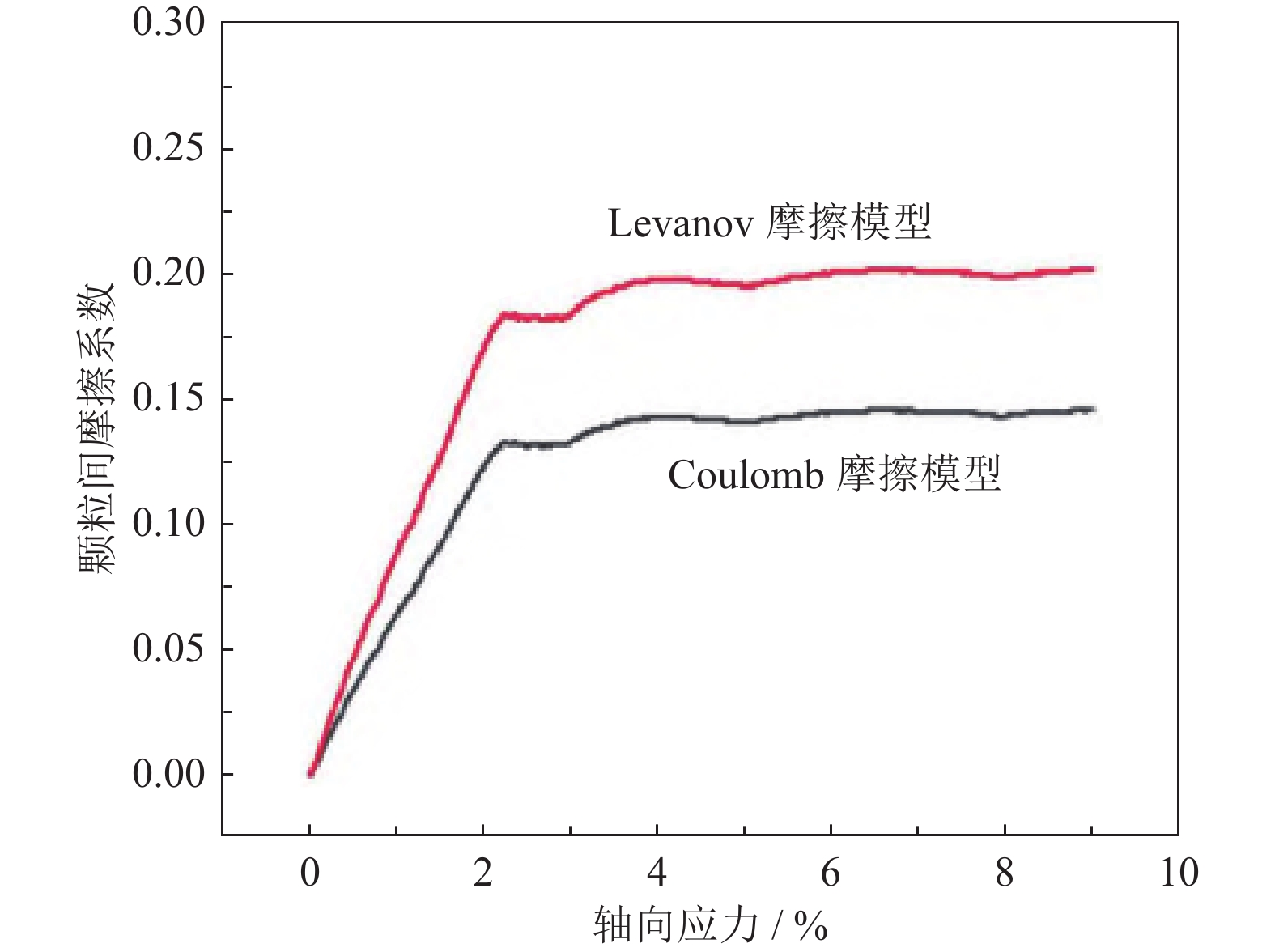
[8] 孟凡净, 刘华博, 花少震, 等. 金属粉末单轴压制过程中的摩擦机制及力学特性分析. 应用力学学报, 2021, 38(3): 1286 DOI: 10.11776/cjam.38.03.B057 Meng F J, Liu H B, Hua S Z, et al. Analysis of friction mechanism and mechanical characteristics of metal powder in the process of uniaxial pressing. Chin J Appl Mechs, 2021, 38(3): 1286 DOI: 10.11776/cjam.38.03.B057
[9] 张炜, 谈健君, 张帅, 等. 基于颗粒物质力学的铁粉末压制中摩擦特性对力链演化影响. 摩擦学学报, 2022, 42(2): 386 Zhang W, Tan J J, Zhang S, et al. Influence of tribological characteristics on the evolution of force chains in ferrous powder compaction based on granular matter theory. Tribology, 2022, 42(2): 386
[10] Zhang N, Zhang S, Tan J J, et al. Correlation mechanism between force chains and friction mechanism during powder compaction. Chin Phys B, 2022, 31(2): 486
[11] Zhang W, Zhang S, Tan J, et al. Investigation on the friction mechanism and its relation to the force chains during powder compaction. J Phys Soc Jpn, 2020, 89(12): 124602 DOI: 10.7566/JPSJ.89.124602
[12] Zhang W, Zhang S, Tan J, et al. Relation between force chain quantitative characteristics and side wall friction behaviour during ferrous powder compaction. Granular Matter, 2022, 24(3): 86 DOI: 10.1007/s10035-022-01244-4
[13] Peters J F, Muthuswamy M, Wibowo J, et al. Characterization of force chains in granular material. Phys Rev E, 2005, 72: 041307
[14] Bassett D S, Owens E T, Porter M A, et al. Extraction of force-chain network architecture in granular materials using community detection. Soft Matter, 2015, 11: 2731 DOI: 10.1039/C4SM01821D
[15] Huang Y M, Daniels K E. Friction and pressure dependence of force chain communities in granular materials. Granular Matter, 2016, 18: 85 DOI: 10.1007/s10035-016-0681-6
[16] 王飞, 刘焜, 王伟. 颗粒的堵塞行为对单向压制过程的影响. 应用力学学报, 2014, 31(3): 400 DOI: 10.11776/cjam.31.03.D028 Wang F, Liu K, Wang W. Simulation of jamming phenomenon of granular matter under single-action pressing process. Chin J Appl Mech, 2014, 31(3): 400 DOI: 10.11776/cjam.31.03.D028
[17] 张炜, 周剑, 于世伟, 等. 双轴压缩下颗粒物质接触力与力链特性研究. 应用力学学报, 2018, 35(3): 530 Zhang W, Zhou J, Yu S W, et al. Investigation on contact force and force chain of granular matter in biaxial compression. Chin J Appl Mech, 2018, 35(3): 530
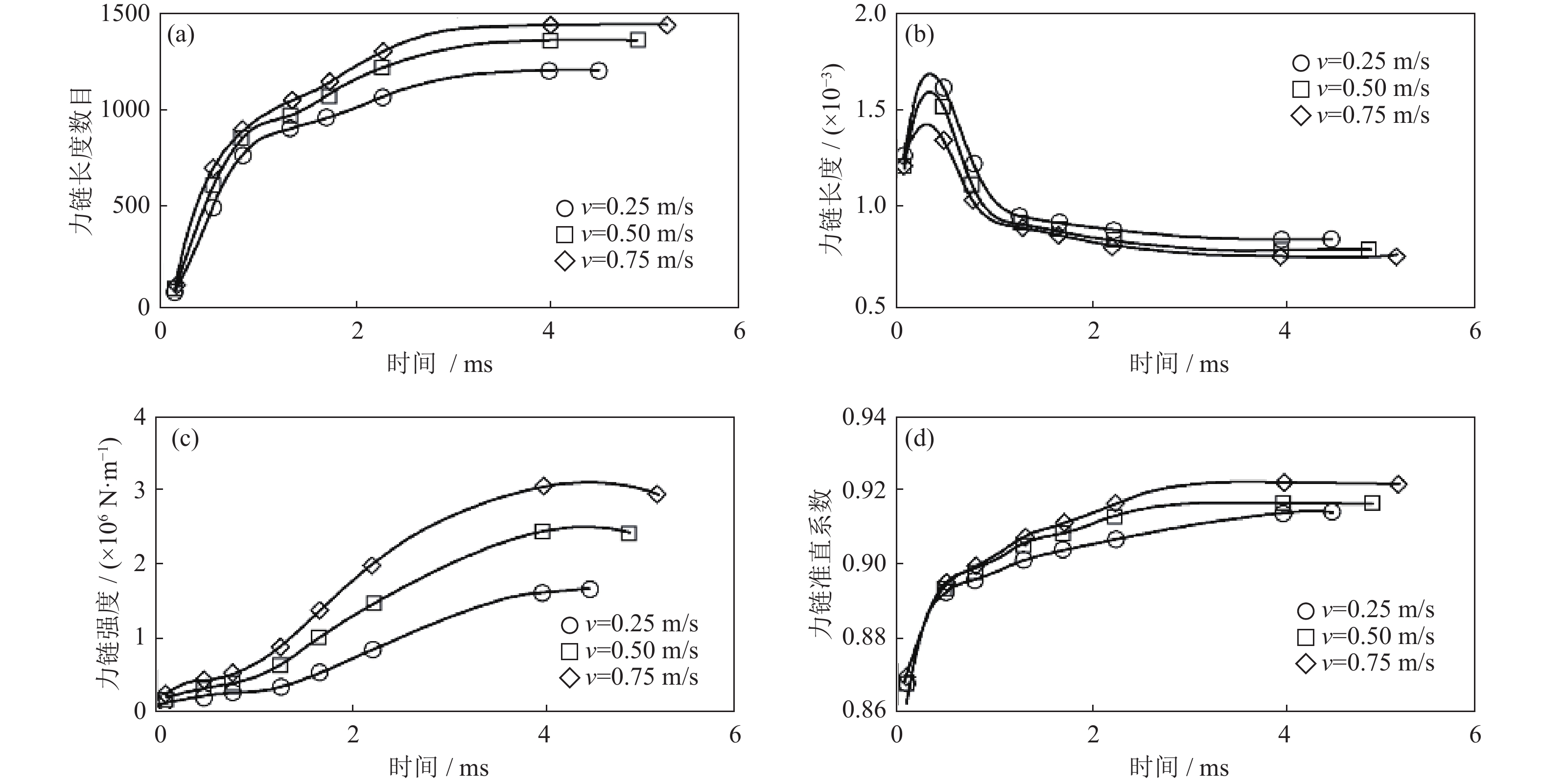
[18] 张超, 刘军, 罗晓龙, 等. 基于离散元法的金属粉末压制加载速度对压力分布影响. 粉末冶金技术, 2019, 37(2): 98 Zhang C, Liu J, Luo X L, et al. Effect of loading speed on pressure distribution in metal powder pressing based on discrete element method. Powder Metall Technol, 2019, 37(2): 98
[19] Zhang L R, Nguyen N G H, Lambert S, et al. The role of force chains in granular materials: from statics to dynamics. Eur J Environ Civ Eng, 2017, 21(7-8): 874 DOI: 10.1080/19648189.2016.1194332
[20] 张炜, 周剑, 于世伟, 等. 基于颗粒物质力学的粉末高速压制过程中应力传递分布分析. 应用力学学报, 2018, 35(1): 154 Zhang W, Zhou J, Yu S W, et al. Investigation of the stress transmission characterization in highvelocity powder compaction based on mechanics of granular materials. Chin J Appl Mech, 2018, 35(1): 154
[21] 张炜, 周剑, 于世伟, 等. 离散元法金属粉末高速压制过程中力链特性量化研究. 机械工程学报, 2018, 54(10): 85 DOI: 10.3901/JME.2018.10.085 Zhang W, Zhou J, Yu S W, et al. Quantitative investigation on force chains of metal powder in high velocity compaction by discrete element method. J Mech Eng, 2018, 54(10): 85 DOI: 10.3901/JME.2018.10.085
[22] 王海陆, 刘军, 林立, 等. 基于离散元的不同粒径配比粉末压制相对密度与力链分析. 粉末冶金技术, 2021, 39(6): 490 Wang H L, Liu J, Lin L, et al. Compacting relative density and force chain analysis of powders with different particle sizes and proportions based on discrete element. Powder Metall Technol, 2021, 39(6): 490
[23] Zhang L, Wang Y J, Zhang J. Force-chain distributions in granular systems. Phys Rev E, 2014, 89(1): 012203 DOI: 10.1103/PhysRevE.89.012203
[24] Gendelman O, Pollack Y G, Procaccia I, et al. What determines the static force chains in stressed granular media? Phys Rev Lett, 2016, 116(7): 078001
[25] Iikawa N, Bandi M M, Katsuragi H. Sensitivity of granular force chain orientation to disorder-induced metastable relaxation. Phys Rev Lett, 2016, 116(12): 128001 DOI: 10.1103/PhysRevLett.116.128001
[26] 李达. 铁基粉末高速压制的数值模拟分析[学位论文]. 合肥: 合肥工业大学, 2014 Li D. Numerical Simulation Analysis of High-Speed Compaction of Iron-Based Powder [Dissertation]. Hefei: Hefei University of Technology, 2014
[27] Peng K F, Pan H, Zheng Z J, et al. Compaction behavior and densification mechanisms of Cu−W composite powders. Powder Technol, 2021, 382: 478 DOI: 10.1016/j.powtec.2021.01.013
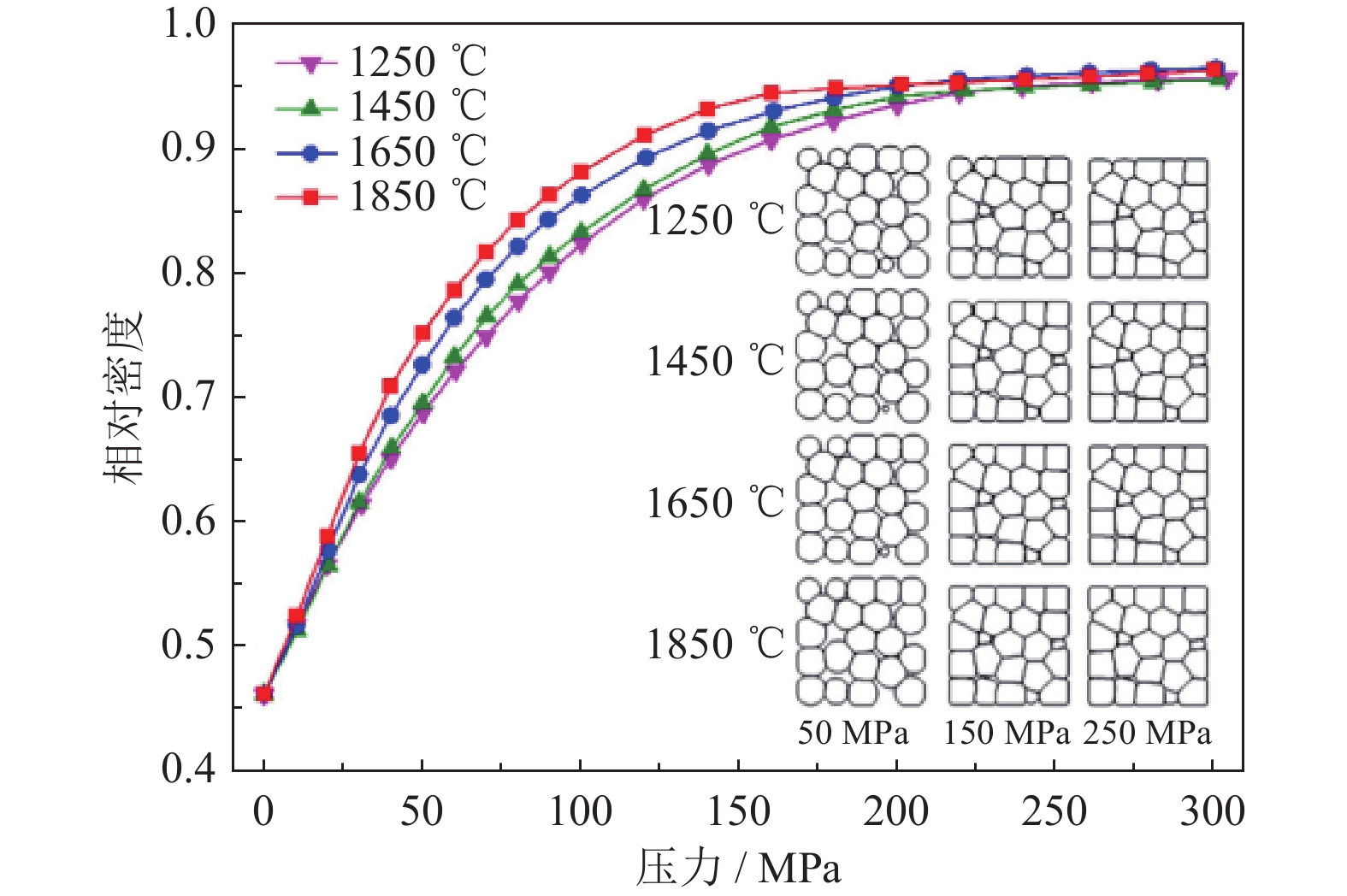
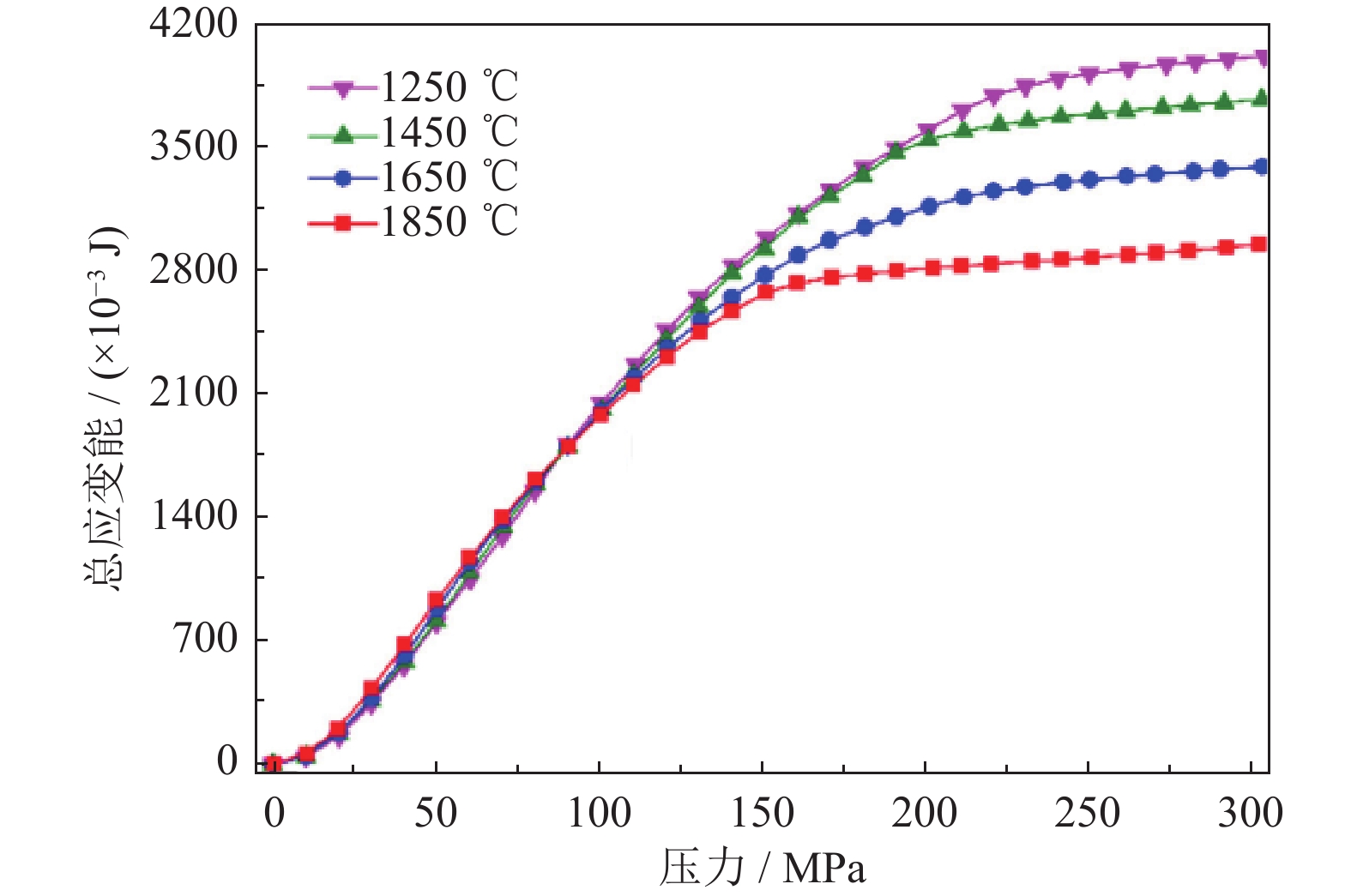
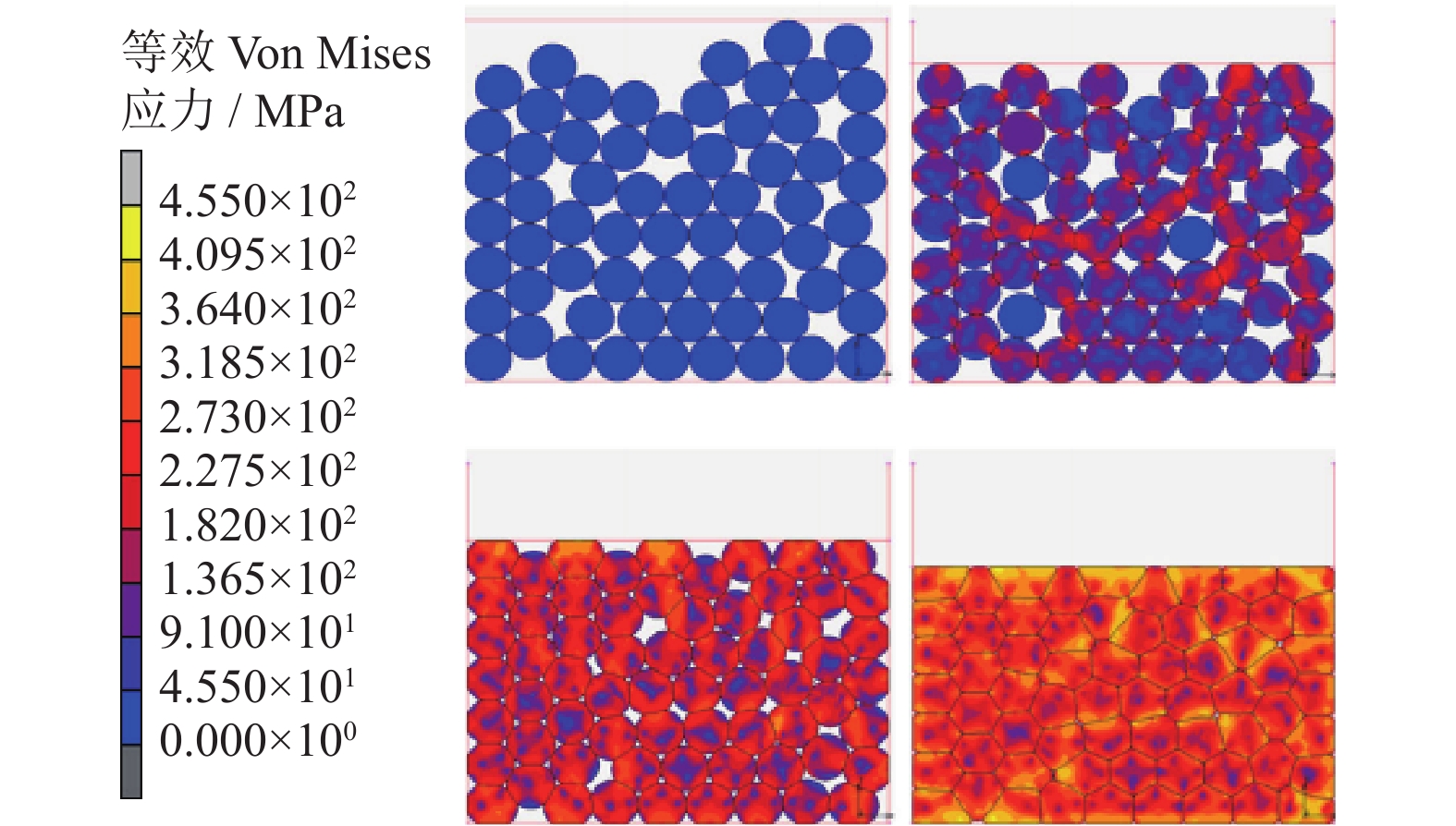
[28] Zhou J, Zhu C Y, Zhang W, et al. Experimental and 3D MPFEM simulation study on the green density of Ti–6Al–4V powder compact during uniaxial high velocity compaction. J Alloys Compd, 2020, 817: 153226 DOI: 10.1016/j.jallcom.2019.153226
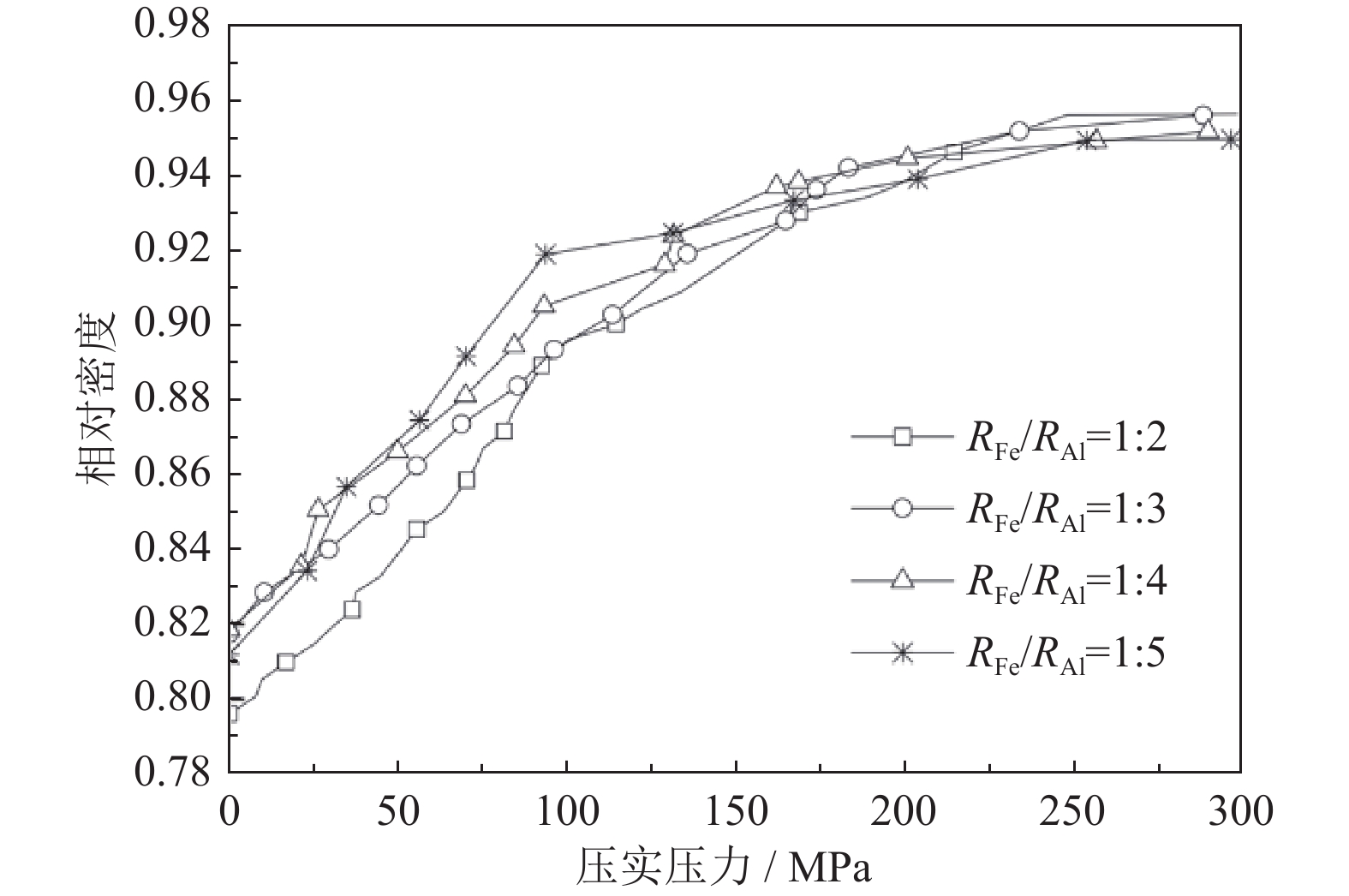
[29] Han P, An X Z, Zhang Y X, et al. Particulate scale MPFEM modeling on compaction of Fe and Al composite powders. Powder Technol, 2017, 314: 69 DOI: 10.1016/j.powtec.2016.11.021
[30] Han P, An X Z, Wang D F, et al. MPFEM simulation of compaction densification behavior of Fe−Al composite powders with different size ratios. J Alloys Compd, 2018, 741: 473 DOI: 10.1016/j.jallcom.2018.01.198
[31] Huang F, An X Z, Zhang Y X, et al. Multi-particle FEM simulation of 2D compaction on binary Al/SiC composite powders. Powder Technol, 2017, 314: 39 DOI: 10.1016/j.powtec.2017.03.017
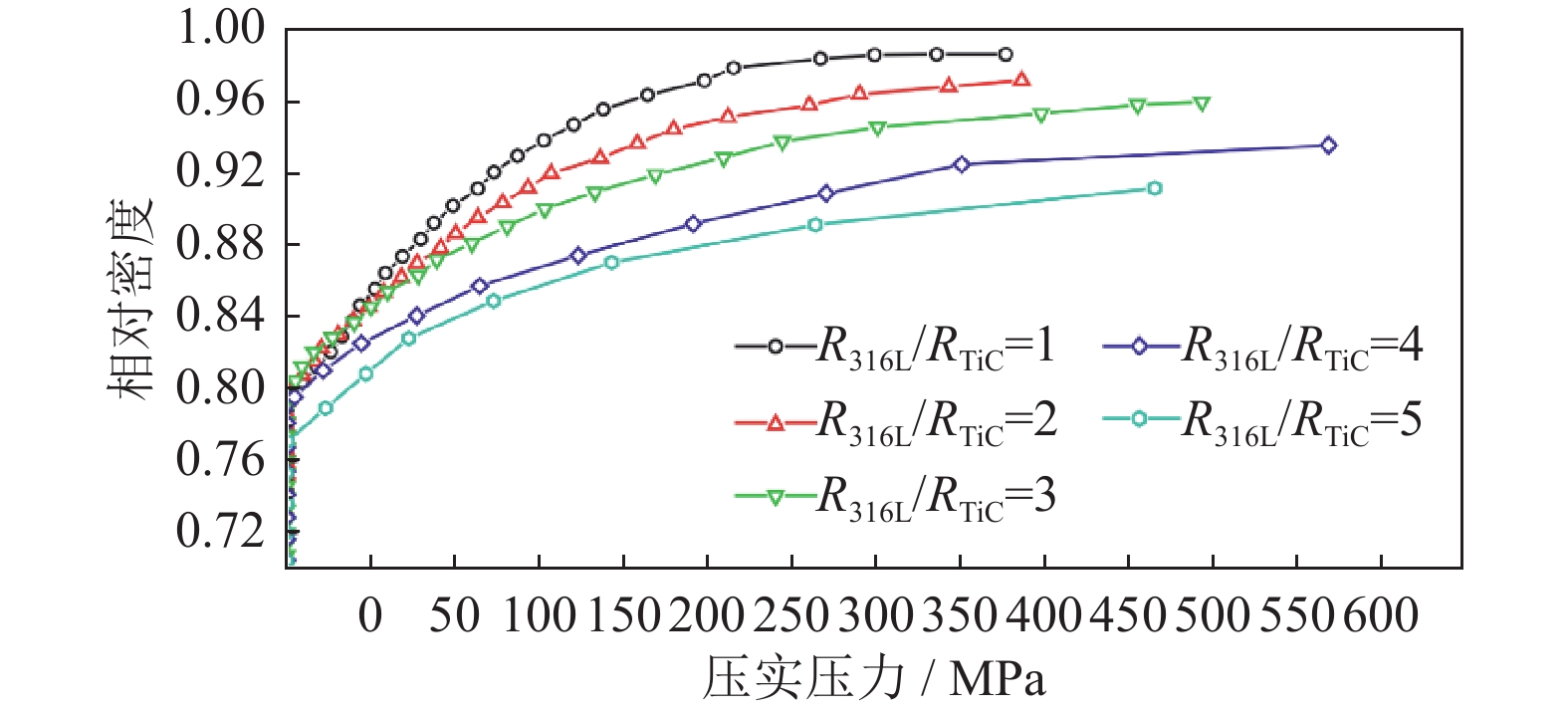
[32] Wang D F, An X Z, Han P, et al. Particulate scale numerical investigation on the compaction of TiC-316L composite powders. Math Prob Eng, 2020, 2020(Pt.6): 5468076.1
[33] Feng Y B, Mei D Q, Wang Y C. Cohesive zone method based multi particle finite element simulation of compaction densification process of Al and NaCl laminar composite powders. J Phy Chem Solids, 2019, 134: 35 DOI: 10.1016/j.jpcs.2019.05.020
[34] Xu L, Wang Y S, Li C Y, et al. MPFEM simulation on hot-pressing densification process of SiC particle/6061Al composite powders. J Phy Chem Solids, 2021, 159: 110259 DOI: 10.1016/j.jpcs.2021.110259
[35] Li M, Lim C V S, Zou R P, et al. Multi-particle FEM modelling on hot isostatic pressing of Ti6Al4V powders. Int J Mech Sci, 2021, 196: 106288 DOI: 10.1016/j.ijmecsci.2021.106288
[36] Jia Q, An X Z, Zhao H Y, et al. Compaction and solid-state sintering of tungsten powders: MPFEM simulation and experimental verification. J Alloys Compd, 2018, 750: 341 DOI: 10.1016/j.jallcom.2018.03.387
[37] Zou Y, An X Z, Zou R P. Investigation of densification behavior of tungsten powders during hot isostatic pressing with a 3D multi-particle FEM approach. Powder Technol, 2020, 361: 297 DOI: 10.1016/j.powtec.2019.08.014
[38] Zhang Y X, An X Z, Zhang Y L. Multi-particle FEM modeling on microscopic behavior of 2D particle compaction. Appl Phys A, 2015, 118: 1015 DOI: 10.1007/s00339-014-8861-x
[39] Zou Y, An X Z, Jia Q, et al. Three-dimensional MPFEM modelling on isostatic pressing and solid phase sintering of tungsten powders. Powder Technol, 2019, 354: 854 DOI: 10.1016/j.powtec.2019.07.013
[40] Loidolt P, Ulz M H, Khinast J. Modeling yield properties of compacted powder using a multi-particle finite element model with cohesive contacts. Powder Technol, 2018, 336: 426 DOI: 10.1016/j.powtec.2018.06.018
[41] Loidolt P, Ulz M H, Khinast J. Prediction of the anisotropic mechanical properties of compacted powders. Powder Technol, 2019, 345: 589 DOI: 10.1016/j.powtec.2019.01.048
[42] Zhou L W, Han P, Liu K, et al. Particulate scale multiparticle finite element method modeling on the 2D compaction and release of copper powder. Math Prob Eng, 2019, 2019(Pt.23): 5269302.1
[43] Güner F, Cora Ö N, Sofuoğlu H. Numerical modeling of cold powder compaction using multi particle and continuum media approaches. Powder Technol, 2015, 271: 238 DOI: 10.1016/j.powtec.2014.11.008
[44] Güner F, Cora Ö N, Sofuoğlu H. Effects of friction models on the compaction behavior of copper powder. Tribol Int, 2018, 122: 125 DOI: 10.1016/j.triboint.2018.02.022
[45] Güner F, Sofuoğlu H. Effects of process parameters on copper powder compaction process using multi-particle finite element method. IOP Conf Ser Mater Sci Eng, 2018, 295: 012027 DOI: 10.1088/1757-899X/295/1/012027
-
期刊类型引用(1)
1. 王娜，武洲，朱琦，席莎，张晓，周莎，李晶，王宇晴. 放电等离子烧结制备钼镍合金. 粉末冶金技术. 2024(04): 361-366 . 
本站查看
其他类型引用(1)
下载:
计量
- 文章访问数: 282
- HTML全文浏览量: 983
- PDF下载量: 72
- 被引次数: 2
