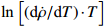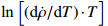First-principles calculation and experimental study on the influence mechanism of diffusion activation energy of Cu atoms in current-assisted sintering
-
摘要:
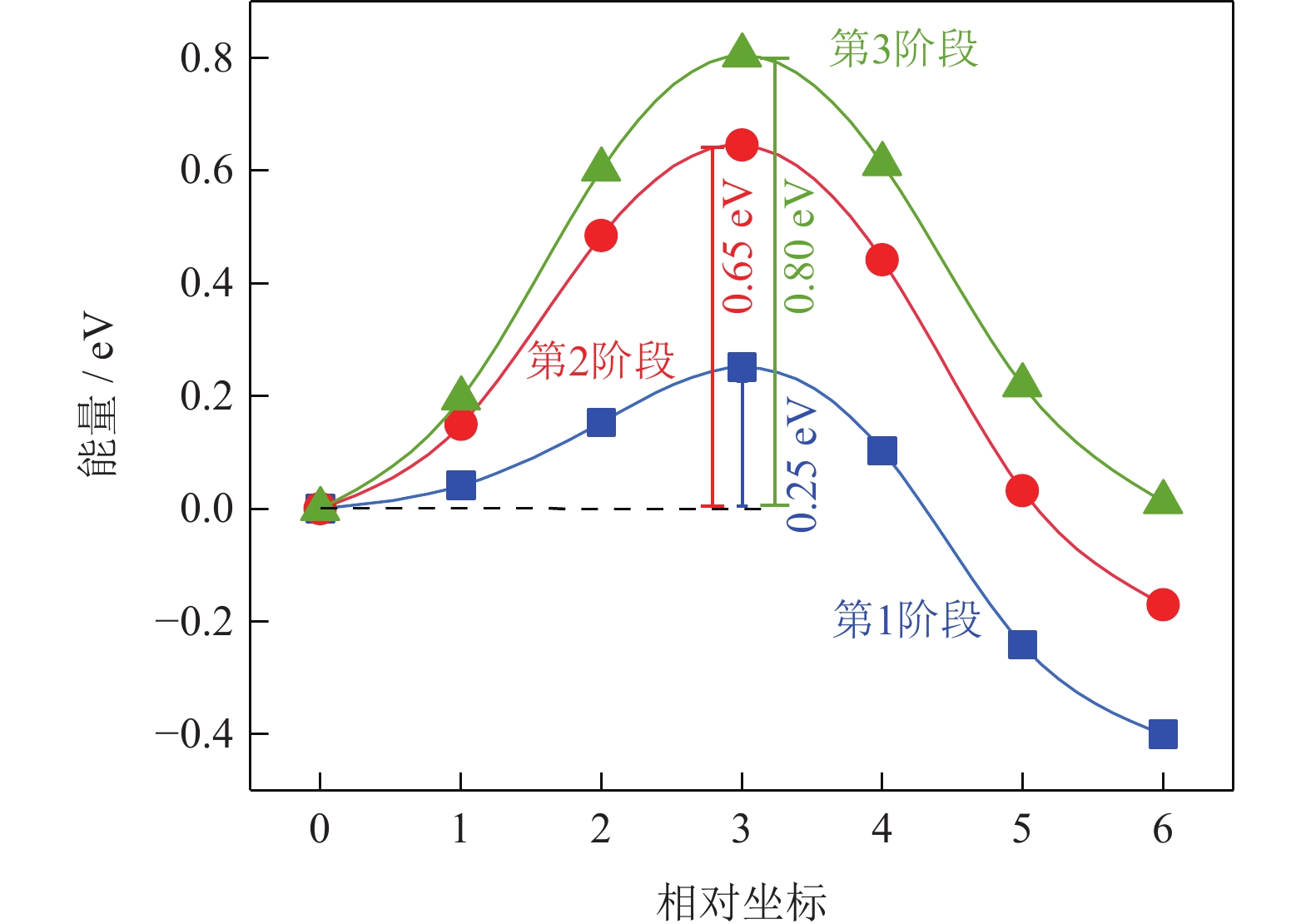
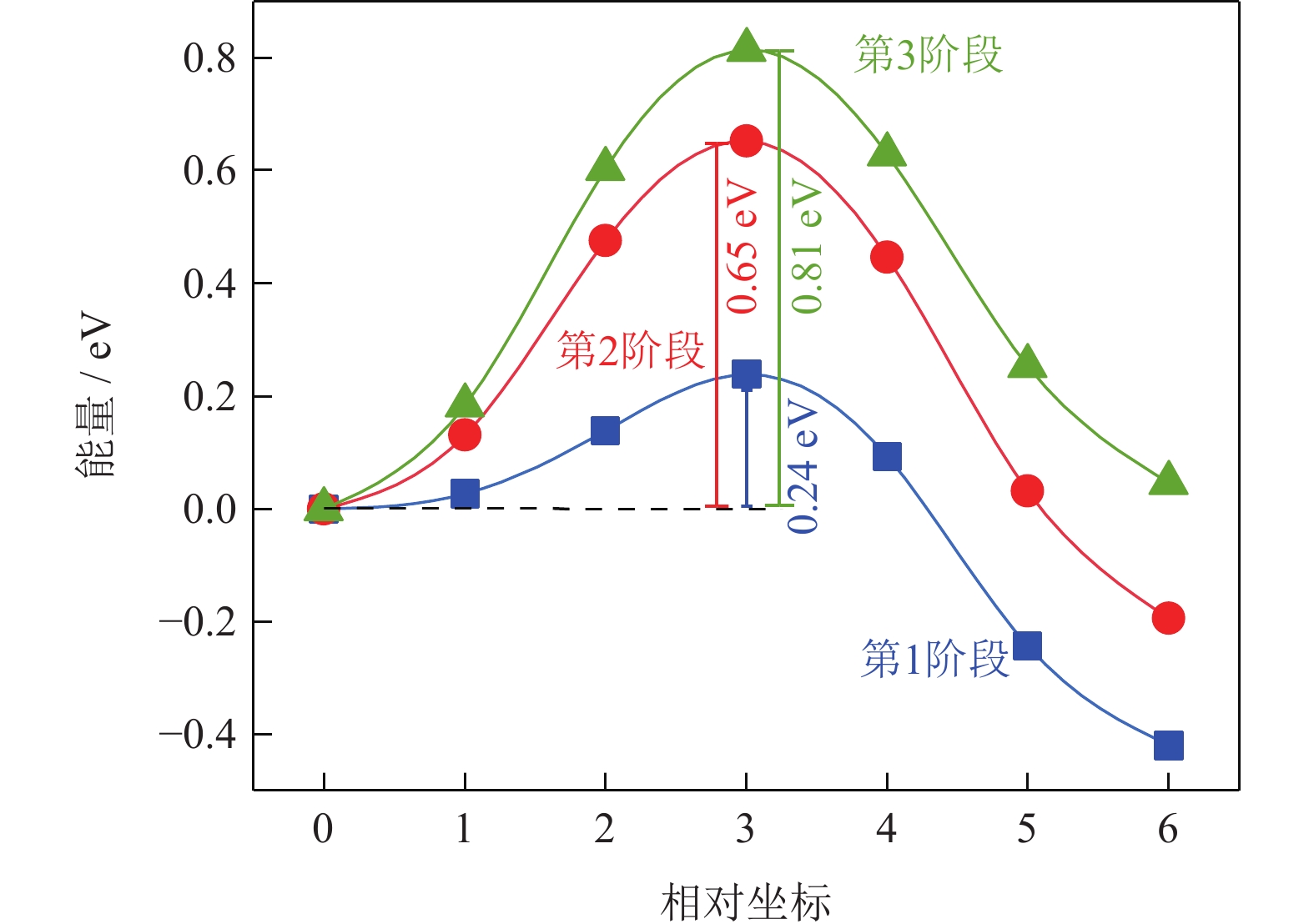
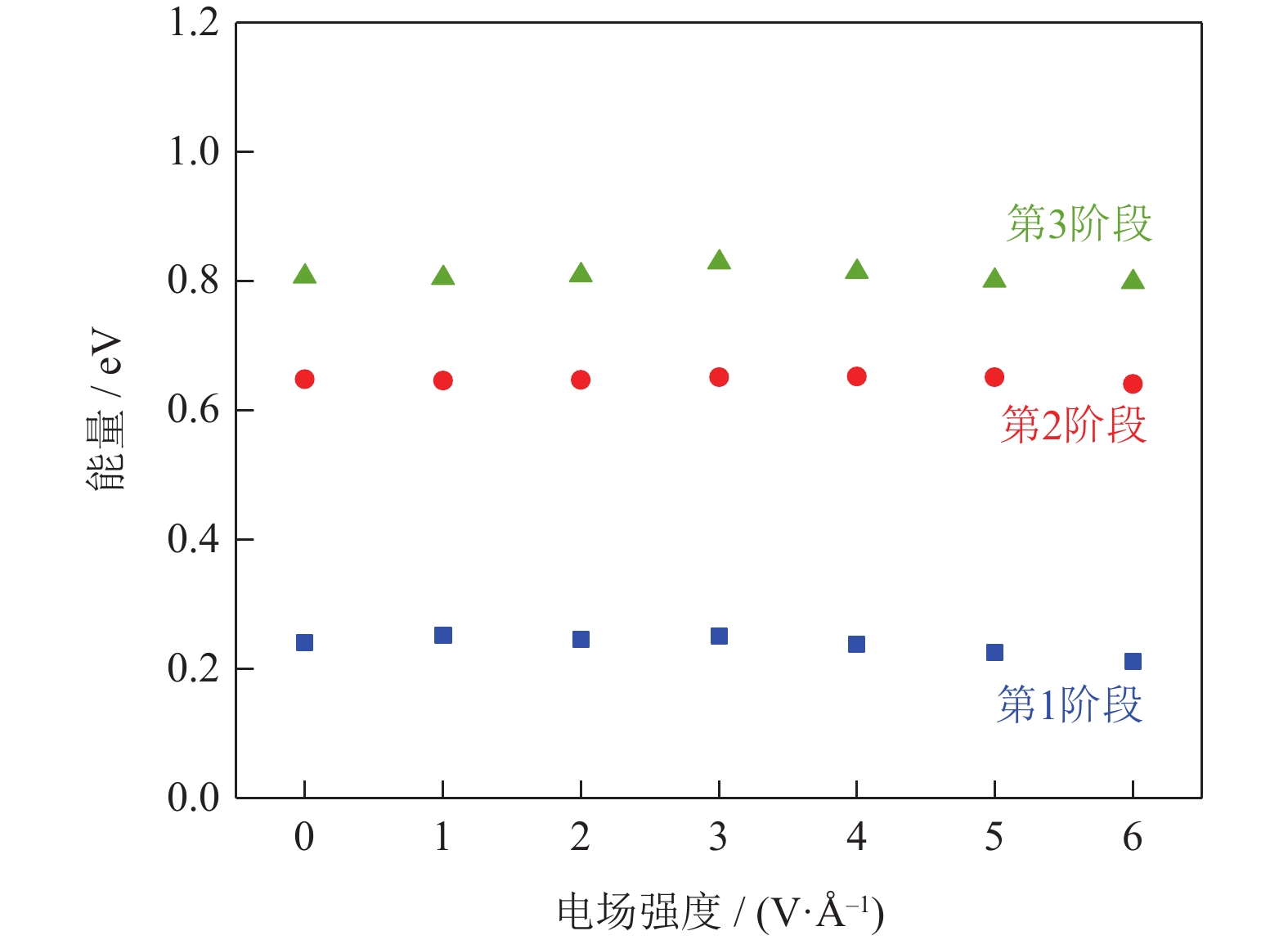
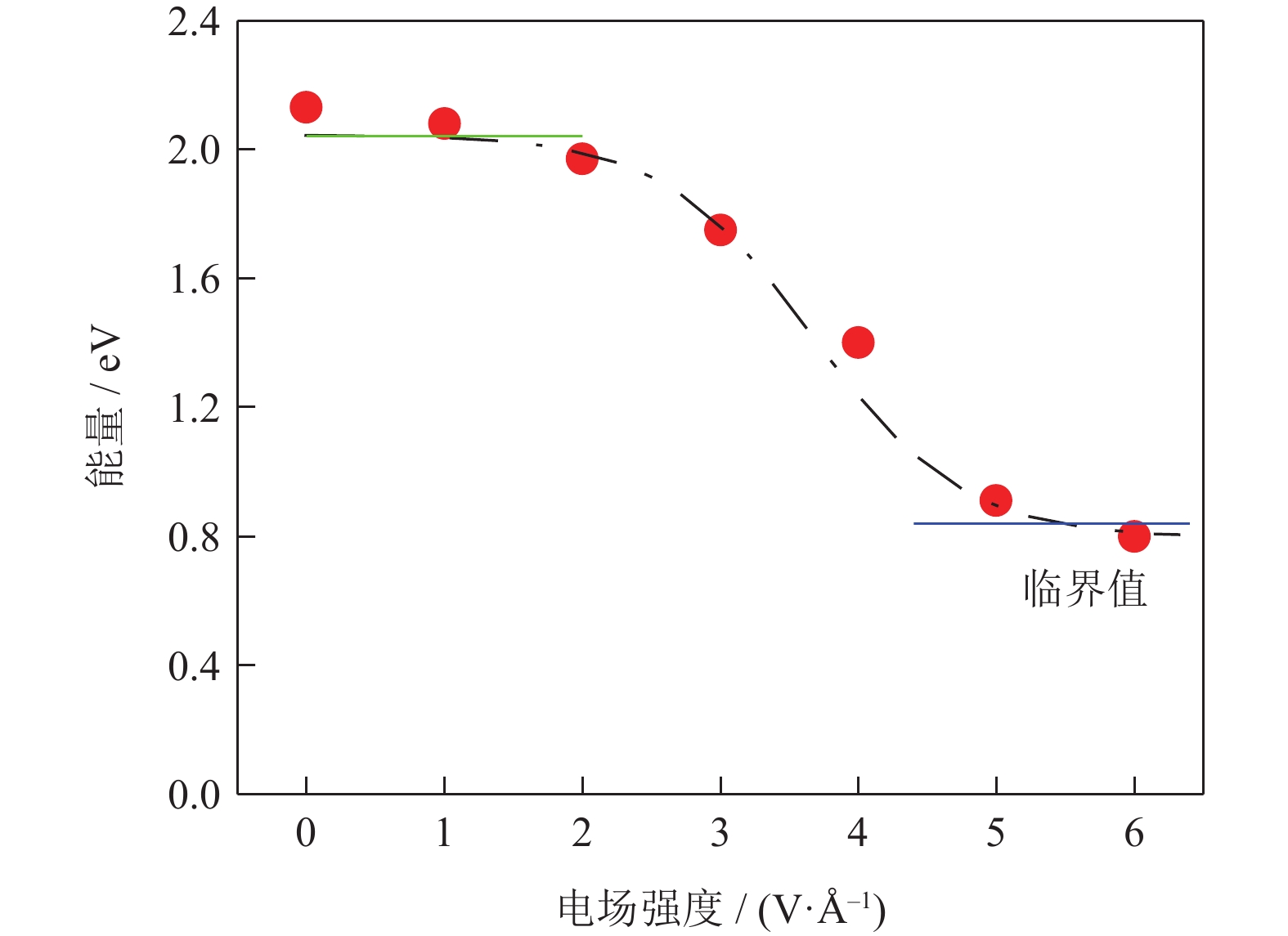
从第一性原理计算与电流辅助烧结两方面出发,研究了外加电场对晶体Cu扩散激活能的影响规律。结果表明,外加电场和电流使Cu空位产生的难度降低,但是原子迁移能几乎不变,导致扩散激活能在达到电场强度(电流密度)阈值(2 V·Å‒1(307.1 A·cm‒2))后略有下降,超过阈值后会剧烈下降,最终在电场强度(电流密度)达到5 V·Å‒1(708.5 A·cm‒2)后,由于空位形成能逐渐下降到0,扩散激活能下降至临界值,扩散激活能临界值相比于阈值对应的扩散激活能降低了约60.2%。研究结果揭示扩散激活能在电场或电流作用下呈现出明显规律性的下降趋势,实验结果与第一性原理计算模拟结果呈现较好的正相关性。
Abstract:The effect of the applied electric field on the diffusion activation energy of Cu crystal was studied by the first-principles calculation and current assisted sintering. The results show that the applied electric field and current reduce the difficulty of Cu vacancy generation, but the atomic migration energy is almost unchanged, resulting in a slight decrease in diffusion activation energy after reaching the electric field intensity (current density) threshold (2 V·Å‒1 (307.1 A·cm‒2)), and a sharp decrease after exceeding the threshold; finally, after the electric field intensity (current density) reaching 5 V·Å‒1 (708.5 A·cm‒2), the vacancy formation energy gradually decreases to 0, the diffusion activation energy drops to the critical value, and the critical value of diffusion activation energy decreases by about 60.2% compared with that of the threshold value. The diffusion activation energy shows a regular decline trend under the action of electric field or current, and the experimental results show a good positive correlation with the first-principle simulation results.
-
-
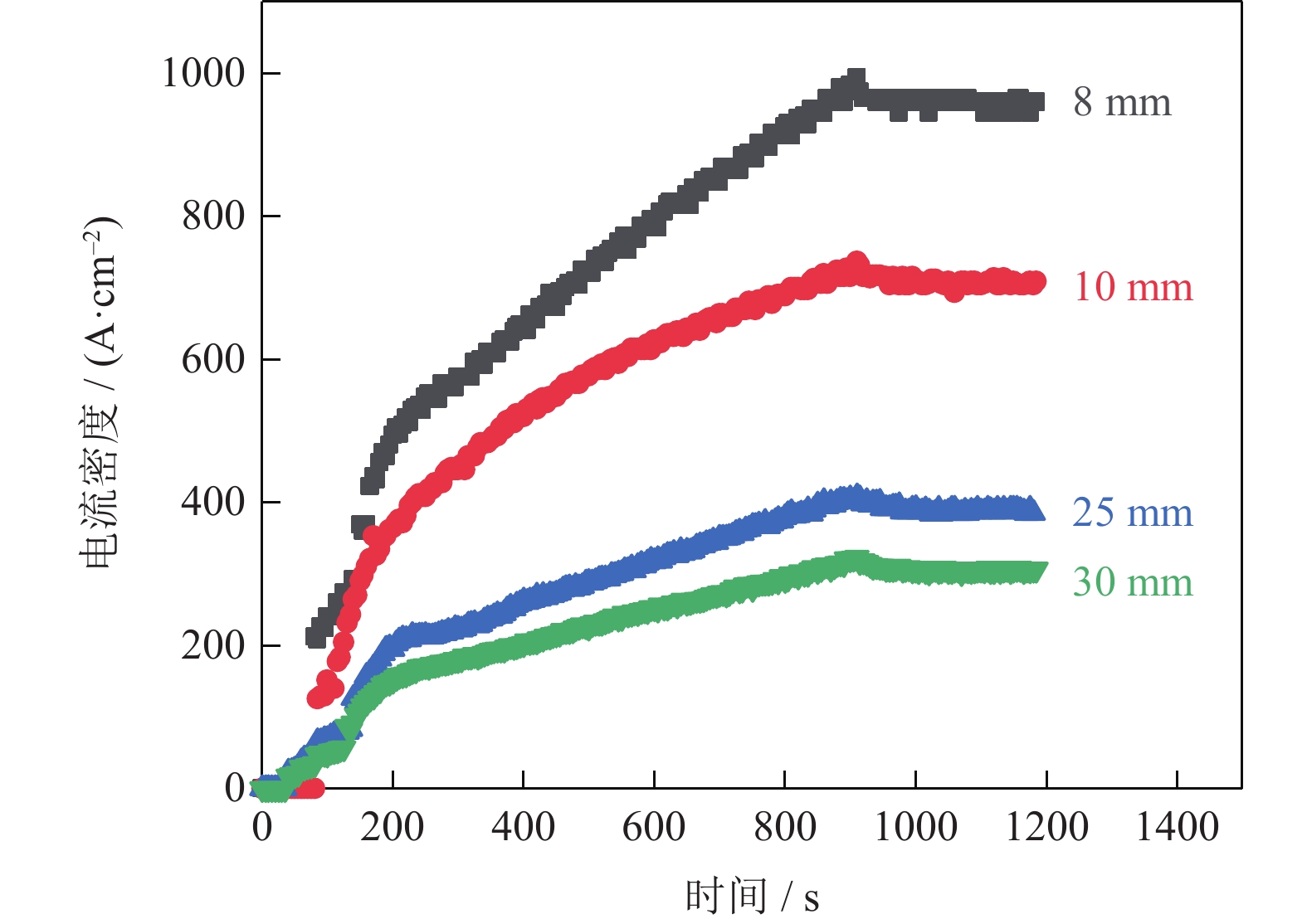
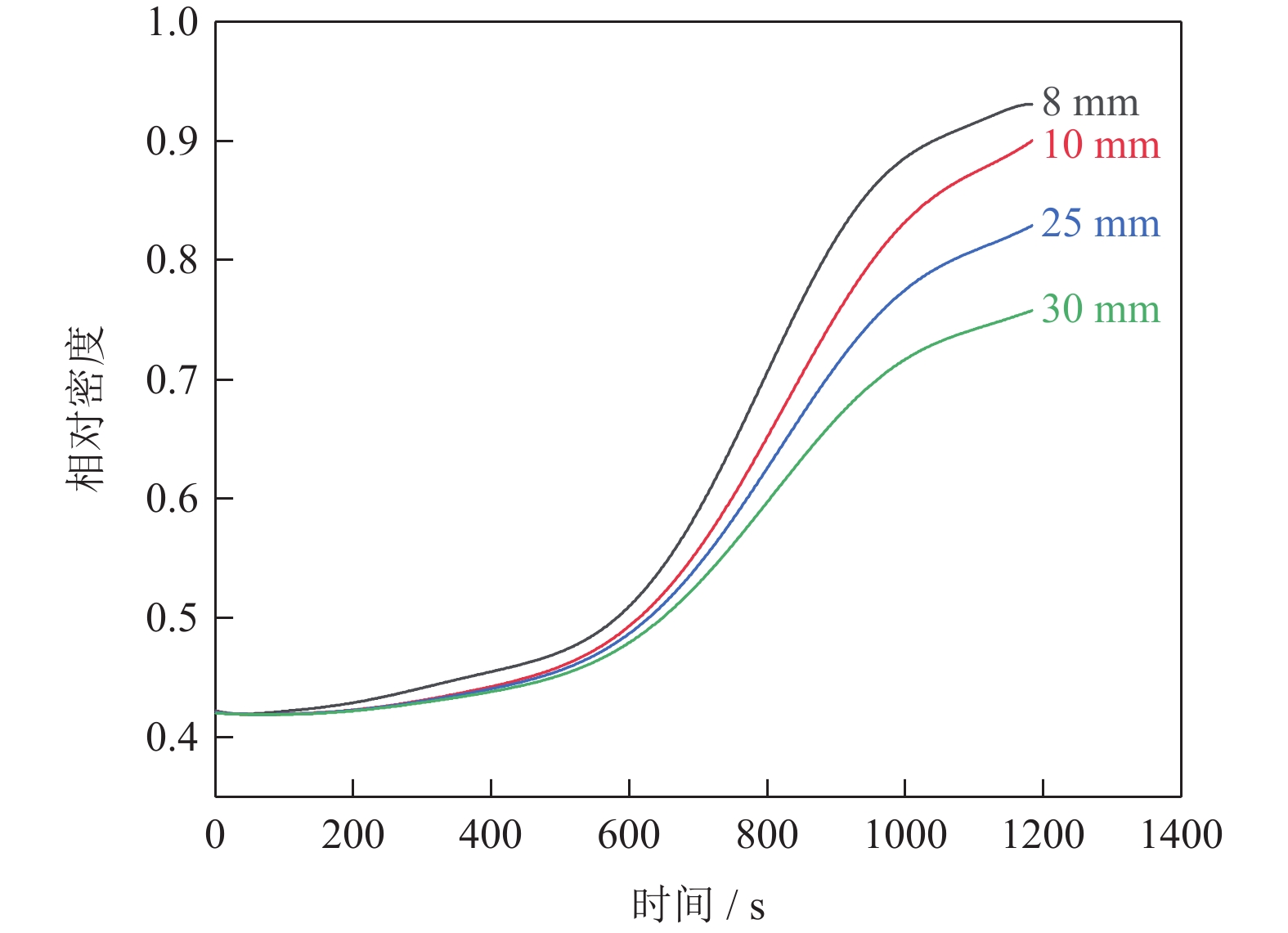
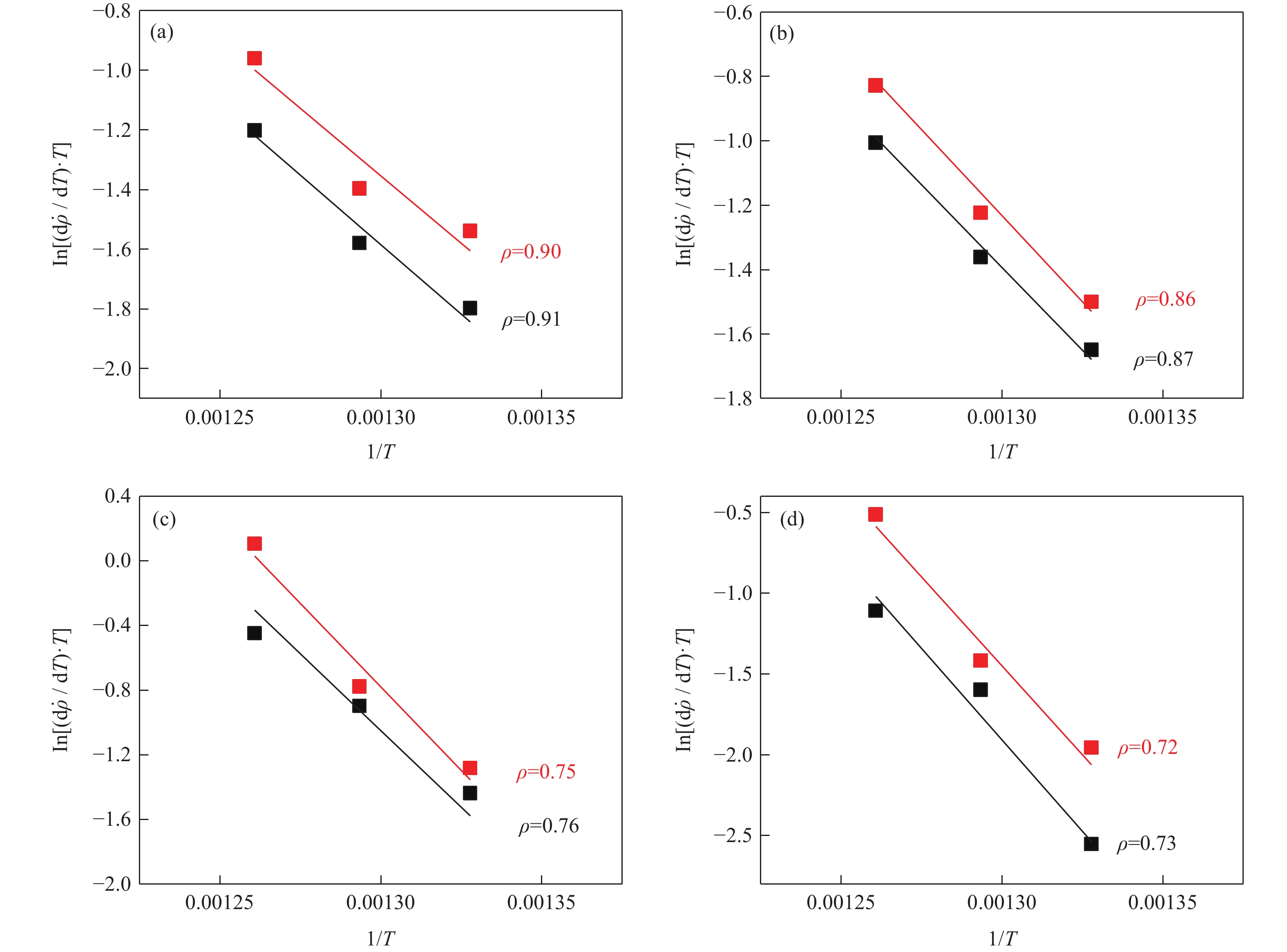
图 15 不同内径石墨模具烧结的
$ {\text{ln}}\left[ {\left( {{\text{d}}\dot \rho /{\text{d}}T} \right) \cdot T} \right] $ 与1/T关系曲线:(a)8 mm;(b)10 mm;(c)25 mm;(d)30 mmFigure 15. Relationship curves of
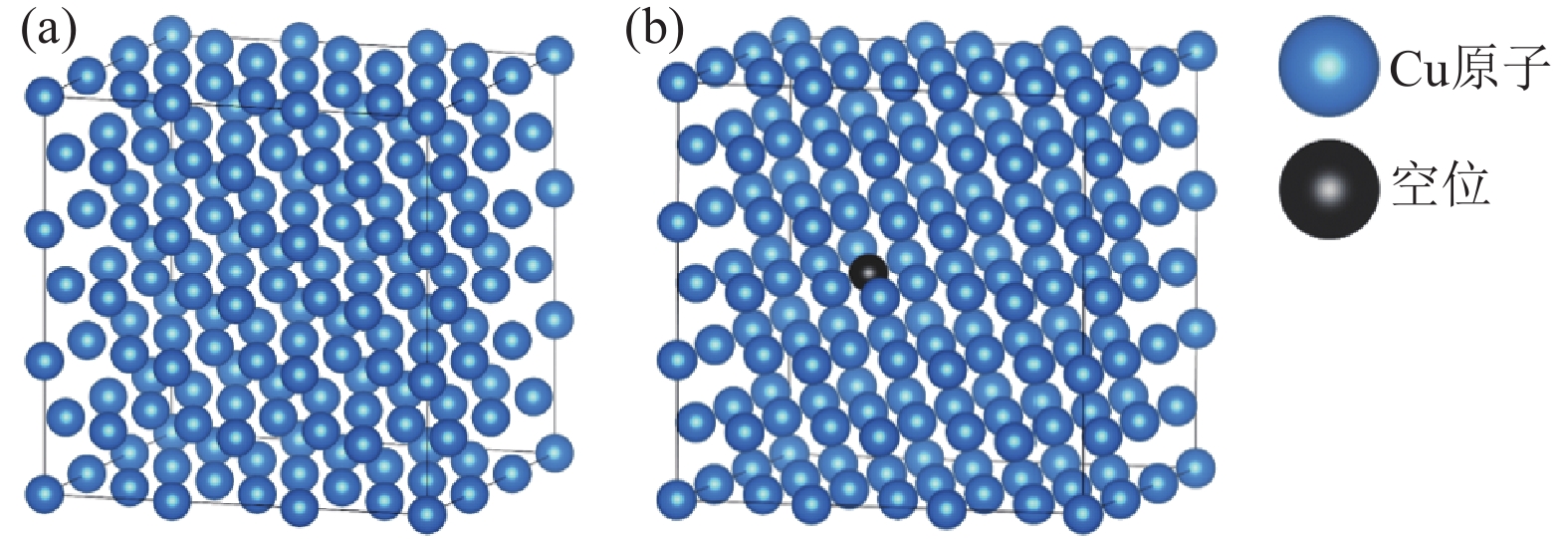
$ {\text{ln}}\left[ {\left( {{\text{d}}\dot \rho /{\text{d}}T} \right) \cdot T} \right] $ and 1/T for the graphite dies with different inner diameters: (a) 8 mm; (b) 10 mm; (c) 25 mm; (d) 30 mm表 1 Cu的空位形成能
Table 1 Vacancy formation energy of Cu
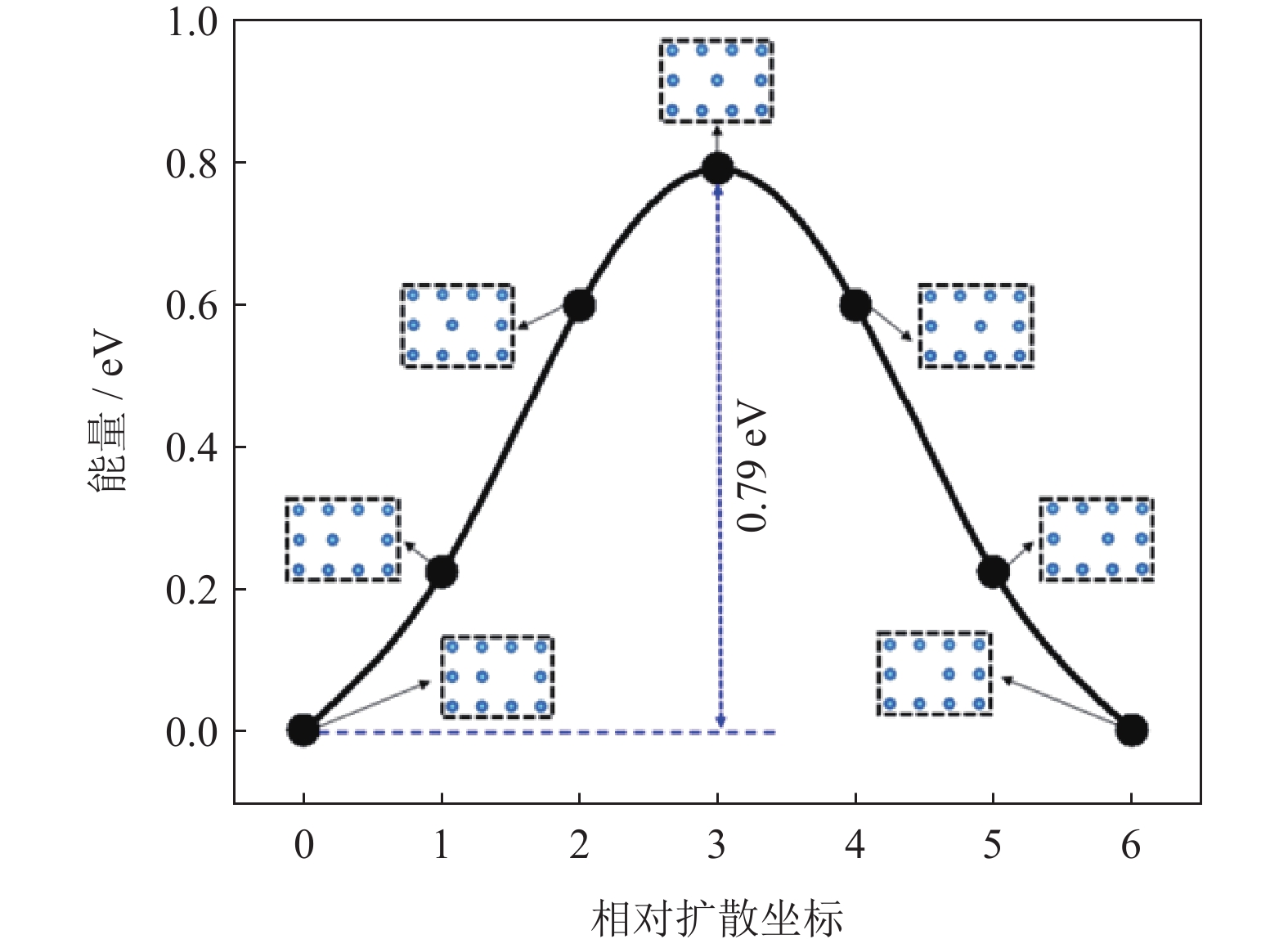
eV EVa EPer EVF ‒397.78 ‒402.83 1.32 表 2 Cu扩散激活能
Table 2 Diffusion activation energy of Cu
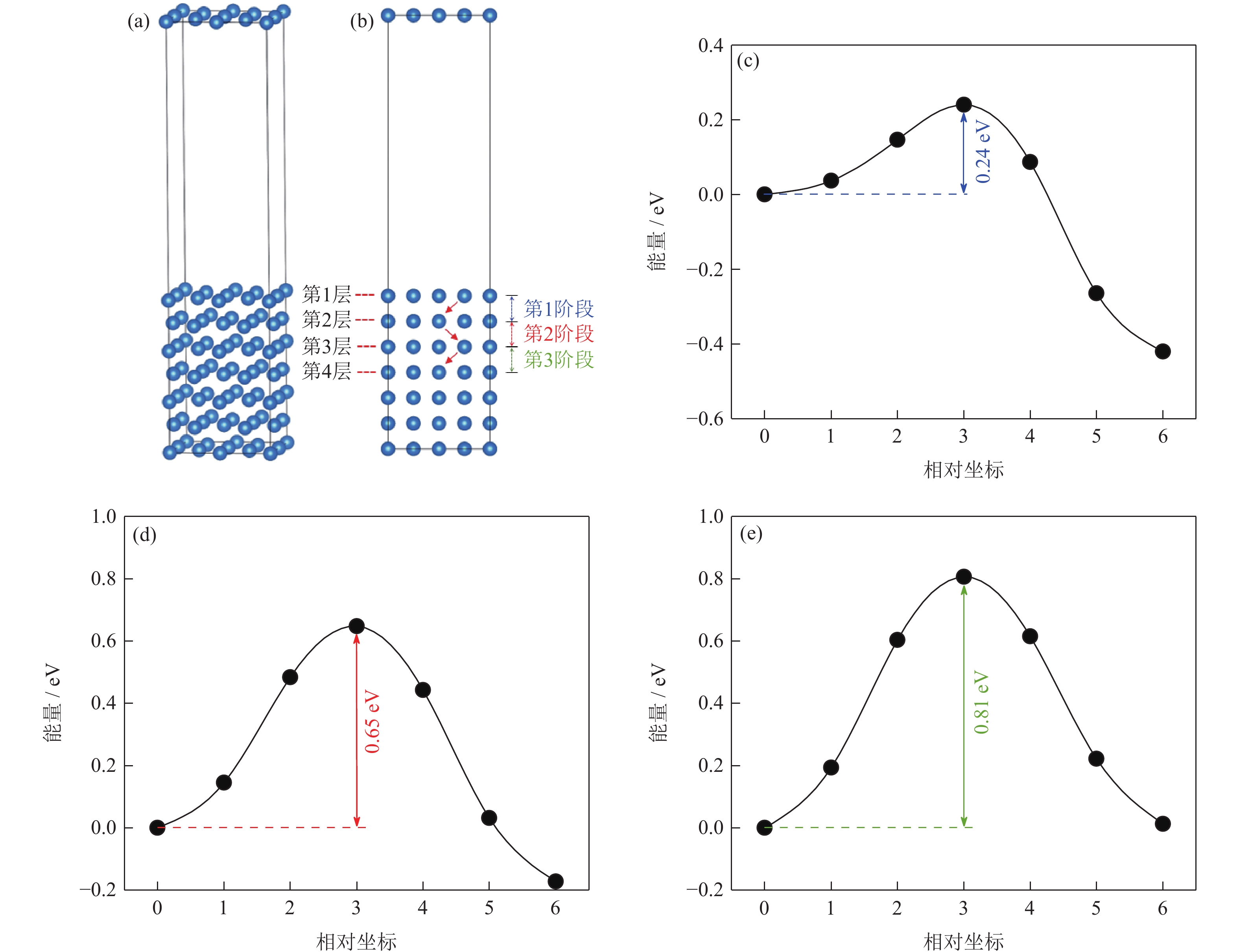
eV 激活能 EVF ESAM EDA 计算值 1.32 0.79 2.11 实验值[23] 1.22±0.02 0.71 2.07 1.29±0.02 0.90 表 3 无电场Cu界面扩散系统各层的空位形成能
Table 3 Vacancy formation energy for each layer of the interfacial diffusion system without electric field
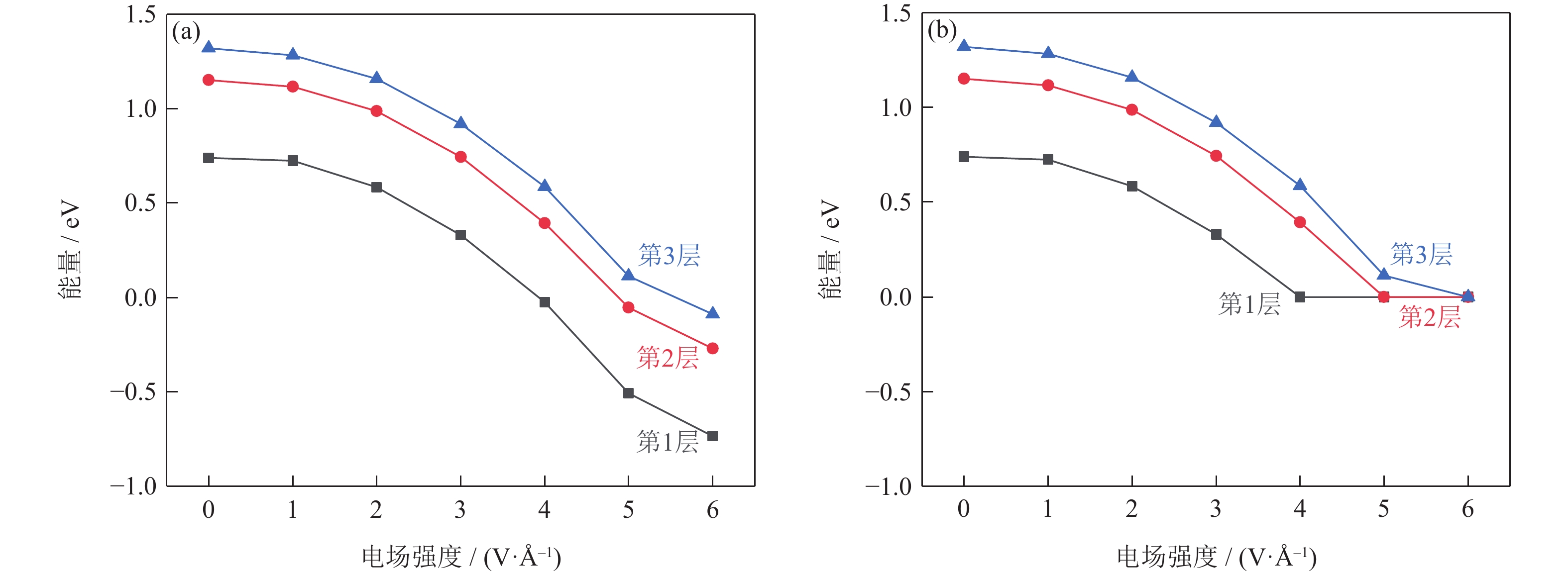
eV Cu界面扩散层 EVa EPer EVF 第1层 ‒195.02 ‒199.32 0.74 第2层 ‒194.61 1.15 第3层 ‒194.44 1.32 第4层 ‒194.44 1.32 -
[1] Choi J, Sung H M, Roh K B, et al. Fabrication of sintered tungsten by spark plasma sintering and investigation of thermal stability. Int J Refract Met Hard Mater, 2017, 69: 164 DOI: 10.1016/j.ijrmhm.2017.08.013
[2] Terentyev D, M Vilémová, Yin C, et al. Assessment of mechanical properties of SPS-produced tungsten including effect of neutron irradiation. Int J Refract Met Hard Mater, 2020, 89: 105207 DOI: 10.1016/j.ijrmhm.2020.105207
[3] Deng S H, Li J N, Li R D, et al. The effect of particle size on the densification kinetics of tungsten powder during spark plasma sintering. Int J Refract Met Hard Mater, 2020, 93: 105358 DOI: 10.1016/j.ijrmhm.2020.105358
[4] Li J, Ding S Y, Zhu J S, et al. Current-density dependence of activation energy in high-Tc superconductor HgBa2Ca2Cu3O8+ x. J Appl Phys, 1995, 77(12): 6398 DOI: 10.1063/1.359113
[5] Garay J E, Anselmi-Tamburini U, Munir Z A. Enhanced growth of intermetallic phases in the Ni–Ti system by current effects. Acta Mater, 2003, 51(15): 4487 DOI: 10.1016/S1359-6454(03)00284-2
[6] Kondo T, Kuramoto T, Kodera Y, et al. Enhanced growth of Mo2C formed in MoC diffusion couple by pulsed DC current. J Jpn Soc Powder Powder Metall, 2008, 55(9): 643 DOI: 10.2497/jjspm.55.643
[7] Deng S H, Li R D, Yuan T C, et al. Direct current-enhanced densification kinetics during spark plasma sintering of tungsten powder. Scr Mater, 2018, 143: 25 DOI: 10.1016/j.scriptamat.2017.09.009
[8] 娄鹤子, 王海滨, 刘雪梅, 等. WC-Co硬质合金摩擦磨损行为的分子动力学模拟. 粉末冶金技术, 2022, 40(5): 8 Lou H Z, Wang H B, Liu X M, et al. Molecular dynamics simulation on friction and wear behavior of WC–Co cemented carbides. Powder Metall Technol, 2022, 40(5): 8
[9] Mukherjee S, Banwait A, Grixti S, et al. Adsorption and diffusion of lithium and sodium on defective rhenium disulfide: A first principles study. ACS Appl Mater Interfaces, 2018, 10(6): 5373 DOI: 10.1021/acsami.7b13604
[10] Yuan Z H, Kong X G, Ma S G, et al. Adsorption and diffusion mechanism of hydrogen atom on the Li2O (111) and (110) surfaces from first principles calculations. J Nucl Mater, 2018, 513: 232
[11] Lu H J, Wu H, Zou N, et al. First-principles investigation on diffusion mechanism of alloying elements in dilute Zr alloys. Acta Mater, 2018, 154: 161 DOI: 10.1016/j.actamat.2018.05.015
[12] Lu C, Yang J, Zhao Y, et al. Influence of applied electric field on atom diffusion behavior and mechanism for W/NiFe interface in diffusion bonding of steel/NiFe interlayer/W by spark plasma sintering. Appl Surf Sci, 2020, 541: 148516
[13] Zhang L Y, Peng C T, Shi J, et al. Surface alloying of chromium/tungsten/stannum on pure nickel and theoretical analysis of strengthening mechanism. Appl Surf Sci, 2020, 532: 147477 DOI: 10.1016/j.apsusc.2020.147477
[14] Kohn W, Sham L J. Self-consistent equations including exchange and correlation effects. Phys Rev, 1965, 140(4A): A1133 DOI: 10.1103/PhysRev.140.A1133
[15] Peng H W, Perdew J P. Rehabilitation of the Perdew-Burke-Ernzerhof generalized gradient approximation for layered materials. Phys Rev B Condens Matter, 2017, 95(8): 081105 DOI: 10.1103/PhysRevB.95.081105
[16] You J S, Fang S A, Xu S Y, et al. Berry curvature dipole current in transition metal dichalcogenides family. Phys Rev, 2018, 98(12): 121109 DOI: 10.1103/PhysRevB.98.121109
[17] Hwang J, Noh S H, Han B. Design of active bifunctional electrocatalysts using single atom doped transition metal dichalcogenides. Appl Surf Sci, 2019, 471: 545 DOI: 10.1016/j.apsusc.2018.11.147
[18] Zhang X, Grabowski B, Körmann F, et al. Accurate electronic free energies of the 3 d, 4 d, and 5 d transition metals at high temperatures. Phys Rev B Condens Matter, 2017, 95(16): 165126 DOI: 10.1103/PhysRevB.95.165126
[19] Kittel C. Introduction to Solid State Physics. 8th Ed. Weenheim: Wiley, 2004
[20] wang Y, Curtarolo S, Jiang C, et al. Ab initio lattice stability in comparison with CALPHAD lattice stability. Calphad, 2004, 28(1): 79 DOI: 10.1016/j.calphad.2004.05.002
[21] Naghavi S S, Hegde V I, Wolverton C. Diffusion coefficients of transition metals in fcc cobalt. Acta Mater, 2017, 132: 467 DOI: 10.1016/j.actamat.2017.04.060
[22] Esfandiarpour A, Nasrabadi M N. Vacancy formation energy in CuNiCo equimolar alloy and CuNiCoFe high entropy alloy: ab initio based study. Calphad, 2019, 66(1): 101634
[23] 王顺花. Cu中空位的计算机模拟. 兰州铁道学院学报, 1997, 16(4): 43 Wang S H. Computer simulation of vacancies in Cu. J Lanzhou Railway Inst, 1997, 16(4): 43
[24] Makov G, Shah R, Payne M C. Periodic boundary conditions in ab initio calculations. II. Brillouin-zone sampling for aperiodic systems. Phys Rev B Condens Matter, 1996, 53(23): 15513
[25] Yu X X, Thompson G B, Weinberger C R. Influence of carbon vacancy formation on the elastic constants and hardening mechanisms in transition metal carbides. J Eur Ceram Soc, 2015, 35(1): 95 DOI: 10.1016/j.jeurceramsoc.2014.08.021
[26] Wang J, Raj R. Activation energy for the sintering of two-phase alumina/zirconia ceramics. J Am Ceram Soc, 1991, 74(8): 1959 DOI: 10.1111/j.1151-2916.1991.tb07815.x
[27] 张剑平, 左红艳, 罗军明, 等. 微波场中铜粉烧结颈形成和晶粒长大动力学. 材料热处理学报, 2018, 39(6): 7 Zhang J P, Zuo H Y, Luo J M, et al. Neek formation and grain growth kinetics of copper powder during microwave sintering. Trans Mater Heat Treat, 2018, 39(6): 7
[28] Demuynck M, Erauw J P, Biest O V D, et al. Densification of alumina by SPS and HP: A comparative study. J Eur Ceram Soc, 2012, 32(9): 1957 DOI: 10.1016/j.jeurceramsoc.2011.10.031
-
期刊类型引用(3)
1. 张炜,萧伟健,袁传牛,张宁,陈荣昕. 基于三维离散元模型粉末压制中力链对阻塞行为的影响机制. 粉末冶金技术. 2024(04): 403-410+417 .  本站查看
本站查看
2. 李化蓥,刘军,张超,张璐栋,王海陆,柯建忠. 基于离散元锥形零件冲击加载的相对密度. 粉末冶金技术. 2023(04): 322-329 .  本站查看
本站查看
3. 张小粉,白瑀,李磊. 基于MARC的FC0205铁基粉末压制模拟技术. 粉末冶金工业. 2023(05): 81-88 .  百度学术
百度学术
其他类型引用(0)



 下载:
下载: